the Creative Commons Attribution 4.0 License.
the Creative Commons Attribution 4.0 License.
Iron oxide inclusions and exsolution textures of rainbow lattice sunstone
Ziyin Sun
Aaron C. Palke
Iron oxide inclusions and exsolution lamellae in rainbow lattice sunstone (RLS) from Harts Range, Australia, are examined using optical and electron microscopy and single-crystal X-ray diffraction (SC-XRD). Laser ablation inductively coupled plasma mass spectrometer (LA-ICP-MS) analyses show a bulk composition of An1.4Ab14.8Or83.0Cn0.8 with < 200 ppmw (parts per million weight) of Fe. Two stages of exsolution can be identified in RLS from the bimodal distribution in the size and shape of the exsolution lamellae. Micron-scaled Albite-twinned oligoclase spindles (An27Ab72Or1) first exsolved at ∼ 650 ∘C were followed by nanoscaled Pericline-twinned albite films (∼ Ab100) below 500 ∘C that create adularescence. The albite films inherited and preserved the monoclinic tetrahedral framework of the orthoclase matrix (An0.3Ab11.5Or87.3Cn0.9) as further ordering was completely inhibited by coherent-interface strain after exsolution. All the exsolution lamellae are pristine and strain-controlled with no signs of any deuteric or hydrothermal alteration, indicating the iron in the magnetite inclusions was not introduced by an external fluid. The magnetite inclusions nucleated around the same time as the exsolution of oligoclase spindles likely due to the reduction of Fe3+ to Fe2+ in the feldspar lattice. Magnetite films following the specific crystallographic orientation relationship (COR) of {111}Mt∥{100}Or and grew to extraordinarily large sizes due to the near perfect lattice match at the interface with the orthoclase host. Some thinner magnetite films were oxidized into hematite during weathering of the host rock. RLS reveals a new mechanism for the formation of the flaky hematite inclusions in feldspars, which may explain the enigmatic origin of aventurescence observed in many other sunstones and red-clouded feldspars.
- Article
(25832 KB) - Full-text XML
-
Supplement
(1851 KB) - BibTeX
- EndNote
Rainbow lattice sunstone (RLS) is a rare gem material that is only found in a pegmatite mine in the Harts Range of Australia, characterized by the perfectly oriented ribbon-like inclusions that produce a spectacular aventurescence effect (Fig. 1a) (Koivula and Gunter, 1989). Magnetite and hematite have been recently identified as the inclusions creating the “rainbow-lattice” effect in RLS (Liu et al., 2018). A strong iridescence (adularescence) effect is also observed in RLS (Fig. 1b) at a slightly different angle from the aventurescence, indicating the presence of submicron, strain-controlled exsolution lamellae (cryptoperthite) in the feldspar crystal. The high potassian composition (Liu et al., 2018) of RLS is very rare, if not unique, for iridescent alkali feldspar because perthites with more potassian bulk compositions (more than Or80) are almost always subject to hydrothermal or deuteric alteration (Parsons and Brown, 1991), which dramatically coarsens the lamellae texture and erases any iridescence effect. This means the RLS is not only a valuable gemstone for its unusual appearance but also a very special mineral specimen with extraordinary thermal and chemical histories.
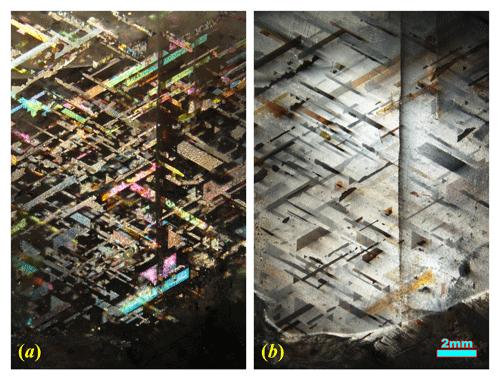
Figure 1The same RLS crystal showing aventurescence (a) and adularescence (b) under reflective light from different angles. Aventurescence is reflection of light from the iron oxide inclusions (dark matrix, bright inclusions), and adularescence is reflection and interference of light from the feldspar (bright matrix, dark inclusions).
The terminology describing the special optical effects commonly observed in feldspars, including such terms as schiller and iridescence, is often poorly defined and confusing. Therefore, it is necessary to be clarified here. All the types of play of light effects in feldspar minerals are created by the reflection of light at a particular angle from inside the feldspar crystal. Two types of reflectors have been identified in feldspars: submicron exsolution lamellae and metallic inclusions with flat shiny surfaces (iron oxide or copper). The effect produced by exsolution lamellae with thickness close to the wavelength of visible light is generally called iridescence in the feldspar literature (Smith and Brown, 1988, p. 20) (although not all iridescent feldspars display rainbow-like colors). These lamellae (<200 nm) can only be observed using an electron microscope as they are thinner than the resolution of an optical microscope. The iridescence in feldspars may appear with different colors depending on the regularity of the exsolution textures. The periodic lamellae in labradorite produce a brilliant play of color (blue to red), known as labradorescence, through constructive interference of light with certain wavelengths. The milky white or silver glow of light (Fig. 1b) observed in moonstones is commonly called adularescence (a misnomer rarely used in the feldspar literature because most moonstones are not adularia). The iridescence in peristerite (sodic plagioclase) may appear similar to either labradorite or moonstone. The reflection from orientated iron oxide or copper inclusions, on the other hand, is called aventurescence (originally “aventurization” by Andersen, 1915). The individual inclusion can be visible under optical microscope and sometimes even to the naked eye (Fig. 1a). The color associated with aventurescence is more complicated. Colors observed can be either the shiny surface color of the opaque inclusions causing aventurescence (red for copper, silver for magnetite) or the thin-film interference color seen for transparent hematite inclusions (Fig. 1a). Gem-quality feldspars with aventurescence are called sunstones (e.g., Oregon sunstone, rainbow lattice sunstone). Aventurescence may appear similar to iridescence when dense micron-scaled inclusions create a homogeneous reflection, and labradorites displaying both iridescence and aventurescence (at different angles) are not uncommon (Jin et al., 2021). The term “schiller” has been used to describe both iridescence and aventurescence (McConnell, 1974; Hofmeister and Rossman, 1983; Ribbe, 1983a; Smith and Brown, 1988), which is a word best known to the gem and mineral collectors but should be avoided in scientific contexts.
Iron oxide inclusions are very common in plutonic feldspar crystals, and they are one of the main causes for the opaque to translucent red or dark color of the otherwise transparent and light-colored mineral. Both the dark clouded feldspars with opaque inclusions and the red-clouded feldspars with transparent hematite inclusions have been extensively studied (e.g., Smith and Brown, 1988, pp. 637–642). All the iron oxide inclusions are distinctively oriented, indicating strong crystallographic constraints from the host feldspar matrix (Copley and Gay, 1979; Nienaber-Roberts, 1986; Sobolev, 1990; Wenk et al., 2011; Ageeva et al., 2020; Bian et al., 2021). The exact mechanism of the formation of these iron oxide inclusions is still poorly understood due to the potentially complex chemical reactions leading to their precipitation and variations in the thermal history and chemistry of the rocks hosting these included feldspars. Exsolution is believed to be responsible (at least partially) for the opaque acicular inclusions, but metasomatism has been advanced as the formation mechanism for the oriented thin flaky inclusions (Smith and Brown, 1988, pp. 637–642). It is possible that the same type of inclusions in various geological settings are formed via different mechanisms despite the similarity in appearances.
Alkali feldspars have been known for their wide varieties of exsolution, twinning and domain micro-textures resulting from subsolidus processes after the initial crystal growth, which can provide a record of the thermal history and replacement reactions of the host rock. The polymorphic and subsolidus phase relations of the alkali feldspars have therefore been intensively studied over the decades given their importance to igneous and metamorphic petrology. The earlier results are best summarized by Brown and Parsons (1989), and the exsolution mechanisms and kinetics are discussed comprehensively by Parsons and Brown (1991). The review by Parsons et al. (2015) includes the more recent microscopy work. The advancement of new technology such as atom probe tomography (APT) has made nanoscaled 3D chemical analysis possible, which provided valuable information for the initial state of the exsolution process (Petrishcheva et al., 2020b). Recent computer modeling has also provided a better understanding of how the lamellae textures are controlled by the cooling history (Abart et al., 2009a, b; Petrishcheva and Abart, 2009, 2012; Petrishcheva et al., 2014, 2020a).
The unique combination of the exsolution lamellae and oriented oxide inclusions in RLS provides a valuable opportunity for understanding the formation of the iron oxide crystals inside feldspars. By combining microscopic observations, chemical analyses (both bulk and microanalysis) and single crystal X-ray diffraction (SC-XRD), the iron oxide inclusions and their relations with the exsolution textures of the host feldspars are studied in this work. A new formation process is proposed for this special case. These results have potentially wide ranging implications for the general forming mechanisms for iron oxide inclusions in feldspars.
RLS is produced from a single source in the world in a pegmatite intruded into the Irindina Gneiss in the Harts Range in the Northern Territory, Australia. The mine is known by various names including the Rainbow Caterpillar mine, Utnerrengatye or Rainbow Serpent Mine. This locality was originally known as a mica mine, under the name Kong mine or Webbs Mica Mine No. 1. The pegmatite consists of fine-grained to medium-grained quartz, orthoclase and muscovite, with some tourmaline and beryl (Rochow, 1962). Graphic intergrowth between quartz and feldspar has also been reported (Thompson, 1986). The limited information about the mining process (https://rainbowlattice.com/mining, last access: October 2021) suggests that the host rock of RLS has been seriously weathered into loose materials, from which the gemstones are hand-mined and screened with large steel sieves. The sample studied in this work is a rough crystal typical of RLS material (2 cm × 1.5 cm × 0.5 cm) acquired from a mineral shop in Alice Springs, Australia.
Pegmatites occur throughout the Harts Range Metamorphic Complex (HRMC), which were emplaced through a series of pulses during the Alice Springs Orogeny (450–300 Ma) (Buick et al., 2008; Scrimgeour, 2013). The pegmatites were not locally derived but fractionated from larger unexposed granite at depth (Buick et al., 2008). The HRMC experienced peak P–T (pressure and temperature) conditions of 8–10 kbar (0.8–1 GPa) and ∼ 800 ∘C, before the Alice Springs Orogeny and the emplacement of the pegmatites at ca. 480–460 Ma (Hand et al., 1999; Mawby et al., 1999; Buick et al., 2001, 2005, 2008). Most of the pegmatites contains perthitic microcline showing strong fluid influences, and orthoclase is very rare (Joklik, 1955a), suggesting that the rock hosting RLS (perthitic orthoclase) is unusually dry in this area. The reported bulk compositions of the perthites from different pegmatites in the HRMC are consistently ∼ An1.5Ab14Or84.5 despite the dramatically different appearances and locations ∼ 100 km apart (Joklik, 1955b).
The chemical composition of the sample was analyzed with a laser ablation inductively coupled plasma mass spectrometer (LA-ICP-MS) at the Gemological Institute of America in Carlsbad, California. A Thermo Scientific iCAP Qc ICP-MS was connected to an Elemental Scientific Lasers NWR213 laser ablation system with a frequency quintupled Nd:YAG laser operated in Q-switched (pulsed) mode at a wavelength of 213 nm and pulse duration of 4 ns. Standard GSD-1G, GSE-1G and NIST SRM 610 were used for external calibration. A 55 µm diameter laser spot size was used to ablate the sample at a 20 Hz repetition rate with a fluence (energy density) of ∼ 10 J cm−2. The composition was initially internally standardized with 29Si at a pre-set value of 248 000 ppmw (parts per million weight). The data were converted to weight percent oxides and normalized to 100 wt %. The concentrations of the major elements are normalized to eight oxygen in feldspar formula as presented in Table 1. The bulk composition of RLS is very similar to those reported for the perthites from other pegmatites in the HRMC (Joklik, 1955b). The concentrations of trace elements are converted back to parts per million weight and listed in Table S1. Some laser ablations spots contain visible iron oxide inclusions, but no obvious increase in the resulting iron concentration is observed.
Table 1Bulk composition of the RLS with major and minor elements from LA-ICP-MS analysis.
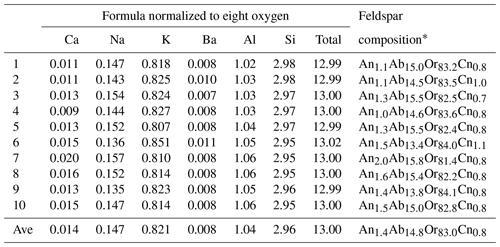
* Or, Ab, An and Cn (orthoclase, albite, anorthite and celsian) represent the K, Na, Ca and Ba end-members in the feldspar composition respectively.
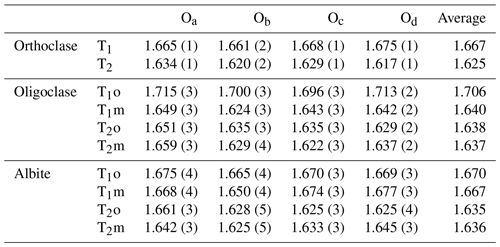
Compositions of individual lamellae and the matrix in the RLS were analyzed with a JEOL JXA-8200 electron probe micro-analyzer (EPMA) equipped with five wavelength-dispersive X-ray spectrometers at the Geological and Planetary Sciences Division Analytical Facility of California Institute of Technology. The data were collected with a focused beam at 10 kV and 10 nA beam current to minimize the interaction volume with the sample. Microcline, albite, synthetic anorthite, benitoite, fayalite and Sr-silicate glass were used as standards to analyze the concentrations of K, Na, Ca, Ba, Al, Si, Fe and Sr. The results of the EPMA analyses are listed in Table 2. The concentrations of Fe and Sr are about the same as the detection limit (dl), and no obvious fractionation between the exsolution lamellae and the matrix was observed. Therefore, Fe and Sr are not listed in the table.
Table 2EPMA results of the spindle lamellae (L2) and the orthoclase matrix (M3), following the notation of Evangelakakis et al. (1993).
Oriented sections parallel to (100), (010) and (001) planes/cleavages of the host feldspar were made in order to best characterize the exsolution lamellae and their relation with the inclusions. Crystals for SC-XRD analysis were cut from the (100) thin section, and the data were collected on a Bruker Apex II Ultra CCD Mo Kα microfocus rotating anode diffractometer at the crystallography lab of the University of California San Diego. The data were collected at 100 K, with the detector set at a distance of 6 cm from the crystal. The CrysAlis Pro software was used to reconstruct undistorted sections of the reciprocal space and refine the lattice parameters from the data, and the APEX3 software was used to integrate peak intensities for structural refinement. The structures were refined using JANA2006 (Petříček et al., 2014), and the 3D structure models and crystal shapes were visualized using VESTA (Momma and Izumi, 2011).
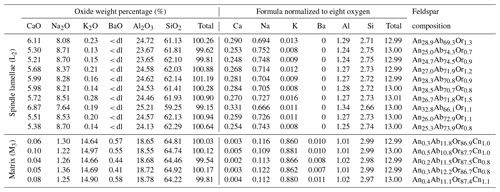
Two different sizes of feldspar exsolution lamellae extended in can be observed under a scanning electron microscope (SEM; Fig. 2). The coarser lamellae (∼2 µm thick) with relatively low aspect ratios are often called “spindles” (Evangelakakis et al., 1993; Abart et al., 2009b), which are regarded as a variety of the film perthite (Parsons et al., 2015). The (010) sections (Fig. 2a, b) of the spindles are more extended with mostly parallel boundaries that taper off quickly near the end, whereas the (001) sections (Fig. 2c, d) show more typical lens shapes. Fractures along the (001) cleavage occur along the spindles as a result of the volume shrinkage of exsolution lamellae relative to the orthoclase matrix. These fractures are enlarged versions of the “pull-aparts” first described by Fitz Gerald et al. (2006). The polysynthetic Albite twins can be directly observed in some larger spindles in the (001) cleavage section (Fig. 2d). Thin films of orthoclase lamellae can sometimes be found “sandwiched” in the spindle lamellae (Fig. 2b), although the 3D topology of this intergrowth cannot be determined. Much thinner film lamellae (∼ 100 nm), matching the expected thickness for the adularescence effect (Fig. 1b) and spreading several tens of micrometers in both [010] and [106] directions, can be found either isolated between the spindles or as “tails” (Tajčmanová et al., 2012) of the spindles (magenta arrows in Fig. 2). The thin films are very similar in dimensions and density as those observed in VSL295 by Evangelakakis et al. (1993). Only the spindles are large enough to be directly observed using an optical microscope, which shows the projections of the lamellae instead of the cross sections as in SEM (Fig. 3). The thin film lamellae are below the resolution of an optical microscope but can produce linear heterogeneous features in the matrix (Fig. 3a, b) through their strain field. The projection perpendicular to plane (Fig. 3c, d) reveals that most of the spindles are belt-shaped (5–10 µm wide and up to >200 µm long) extending in the [106] direction (Fig. 3c), whereas irregular shapes with pointy ends and zigzag edges also sporadically occur (Fig. 3d).
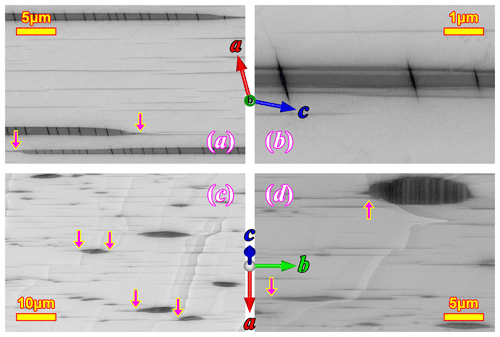
Figure 2SEM images of the (010) section (a, b) and the (001) section (c, d) showing the feldspar exsolution lamellae in RLS. Much thinner films (∼ 100 nm), which are responsible for the adularescence effect (Fig. 1b), can be observed in between the larger spindles. The arrows marks some of the thin films appearing as “tails” rooted in the larger spindles.
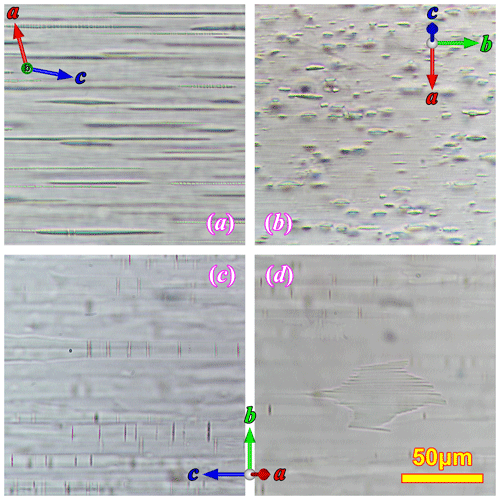
Figure 3Plagioclase exsolution lamellae in the RLS under optical microscope in plane polarized light. The crystallographic orientation of the orthoclase matrix is marked on each image.
The compositions of the different components in the RLS are labeled following the same notation as Evangelakakis et al. (1993): L2 for the coarse spindles, L3 for the thin films, and M2 and M3 for the matrix in between the coarse spindles (including thin films) and the matrix between thin films (excluding all exsolution lamellae) respectively. The thin films are much smaller than the interaction volume of the electron beam; therefore only the pure-phase composition of L2 (spindles) and M3 (matrix excluding all exsolution lamellae) can be accurately analyzed with EPMA. The coarse spindles (L2) are mostly oligoclase with compositions between An25 and An30 (Table 2). More calcic compositions up to ∼ An33 may occur in the center of some larger irregular blebs. Very low K (∼ Or1) and no Ba (below detection limit) are detected in L2. The orthoclase matrix (M3) is quite homogeneous with low Ca concentration (An0.3Ab11.5Or87.3Cn0.9). The M2 composition (weighted average of L3 and M3) should be only slightly more sodic than M3 given the small volume proportion of thin film lamellae (L3) in the matrix.
The iron oxide inclusions in the RLS (Fig. 4) can be easily distinguished by their body color. Most of the inclusions are dark-colored magnetite crystals, and only a small proportion of them are light-colored hematite. Hematite often appears as irregular patches on the edges or corners of the thinner magnetite crystals, indicating that they are secondary to the magnetite crystals resulting from oxidation. The thickness of the iron oxide inclusions measured from SEM images (Fig. S1 in the Supplement) are all less than 200 nm, agreeing with the colors of the hematite inclusions (both the body colors and the interference colors as shown in Fig. S2) and the slight transparency of the magnetite inclusions (Yazdi et al., 2011). Therefore, these thin oxide inclusions will be referred to as “iron oxide films” (or “magnetite films” and “hematite films”) in this paper.
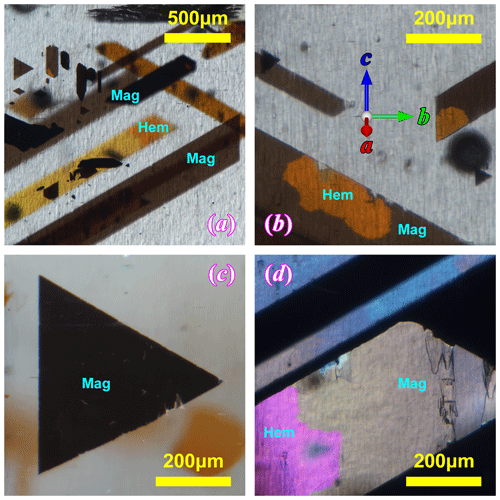
Figure 4The thin film iron oxide inclusions in the RLS under optical microscope. All the images are taken with the same orientation of the orthoclase as labeled in (b). Panels (a), (b) and (c) are bright-field-transmission illuminated, and (d) is taken under reflective lighting. Magnetite appears black or dark brown, and hematite appears light yellow to deep orange in transmission light (a–c). The pink color in the bottom left corner of (d) is the interference color of a hematite patch in a magnetite-dominated film, similar to the one shown in (b). The blueish color of the narrower inclusion in (d) is tarnishing on a magnetite film, which is also common in the RLS.
Most of the iron oxide films appear as bands extended at an angle ∼ 60∘ relative to the (010) plane (Fig. 4). The film inclusions cover a wide range of sizes, from ∼ 100 µm to 5 mm in length. The widths of the bands, however, rarely exceed 500 µm (Fig. 1). The corners of these flakes form angles of either 60 or 120∘, indicating a hexagonal crystal form. It is also very common to find smaller inclusions with a perfect equilateral triangle shape with one edge parallel to the (010) plane (Fig. 4c). These shapes suggest that all the iron oxide films started growing as symmetrical triangles or rhombi until one pair of the edges got abandoned, and growth only occurred along the other direction.
The relation between the iron oxide films and the oligoclase spindles is better shown in the (010) sections (Fig. 5). The oxide films are exactly perpendicular to the (010) plane as their projections only appear as thin lines. The oxide films are viewed along their longest dimensions, which extend along the entire length of the section. By measuring the angles among the oxide films, the (001) cleavage and the oligoclase spindles, most oxide films are seen to be parallel to the (100) plane of the orthoclase matrix, and some oxide films are found to be parallel to the (102) planes of the orthoclase. All of the (102) oxide films appear to cut across the oligoclase spindles (Fig. 5a, b), and some slightly smear the oligoclase spindle along the oxide film (Fig. 5b). This cross-cutting relation indicates that the iron oxide films grew after or during the final stage of the oligoclase spindle growth. The (100) oxide films, on the other hand, appear to have formed earlier. They either have no intersections with the oligoclase spindles (Fig. 5e) or strongly affect the shapes of the oligoclase spindle that they intersect with (the blebs in the oligoclase spindles shown in Figs. 5c and d and S1d are only found at intersections with the film inclusions), suggesting that they grew before or during the early stage of the spindle growth.
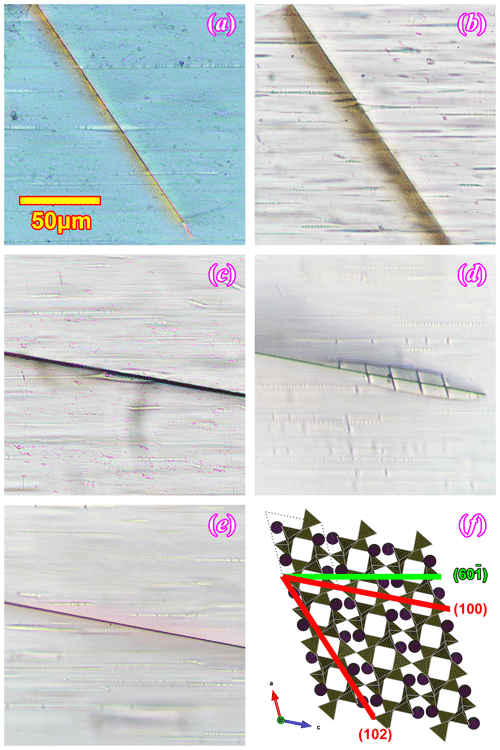
Figure 5The film inclusions in the (010) sections of the RLS under optical microscope. Panel (a) is taken between crossed polarizers, (b) and (c) are under plane polarized light, and (d) and (e) are under focused plane polarized light with thicker sections. The spatial relationship between the orthoclase structure, the oligoclase spindle (subparallel to ) and the two iron oxide film orientations ((100) and (102)) is illustrated in (f).
A different type of magnetite inclusion (identified with SC-XRD in the next section), which will be referred to as the “magnetite seeds” in this paper, can be found in the RLS at higher magnification under the optical microscope (Fig. 6). The magnetite seeds are sparsely dispersed throughout the crystal and are hard to notice at lower magnifications. However, they are somewhat common and can be found without much difficulty when looked for intentionally. These crystals have much lower aspect ratios compared to the ribbon-like thin film inclusions. The euhedral isometric crystal form (cubic or octahedral) can be identified on some of the seeds. They all are about the same size, ∼ 10 µm in diameter. The magnetite seeds appear to be randomly oriented relative to the orthoclase host. All of these seeds are accompanied by a larger irregular-shaped oligoclase spindle similar to the one in Fig. 2d, which does not completely engulf the magnetite seeds but is always in direct contact with part of the crystal. A tiny particle (∼ 1–2 µm) of an unidentified phase can sometimes be found in the albite lamella associated with the magnetite seed (marked by blue arrows in Fig. 6b–f).
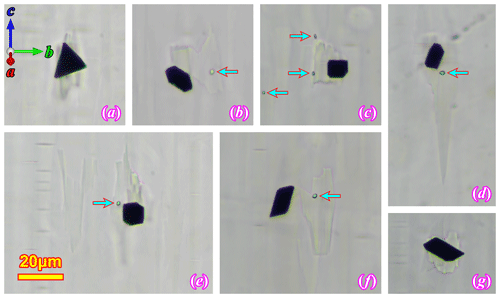
Figure 6Magnetite seeds in (100) thin sections under optical microscope. All the images are taken under plane polarized light. Panels (c), (d) and (e) are focus-stacked to bring both the magnetite crystal and the associated albite lamellae into focus. The arrows mark an unidentified phase that is commonly associated with the magnetite seed and oligoclase spindle.
Two crystals are analyzed with SC-XRD: one containing a magnetite film (Crystal 1) and the other a magnetite seed (Crystal 2). Four different phases (three feldspars + magnetite) and up to eight domains can be identified in the SC-XRD data. Some experimental details are summarized in Table 3, and the T–O bond distances of the refined structures are listed in Table 4. The crystallographic information file (CIF) of the refined structures can be found in the Supplement, which contains the complete and detailed description of the data reduction and structure refinement process for the significantly overlapped data.
The reconstructed sections of the reciprocal space of some major orientations of Crystal 1 are shown in Fig. 7, with the reciprocal lattice of orthoclase, oligoclase, albite and magnetite marked by dashed lines. Orthoclase is evidently the dominant phase, with the diffraction peaks much stronger than the other phases. The average structure of orthoclase is of monoclinic symmetry with space group. The “star-shaped” streaking around Bragg peaks (Fig. 7c) indicates a typical “tweed” structure containing nanoscaled twin domains with short range Al–Si ordering in the T1 sites (Brown and Parsons, 1989). Very diffuse scattering centered on the , violating the C-centering condition, can be observed in the h2l section as shown in Fig. 8a, which is evidence for local K–Na ordering resulting from slow cooling at low temperature (Xu et al., 2019). The lattice parameters of the orthoclase structure plot are very close to the unstrained band in the diagram proposed by W. H. Bernotat (Kroll and Ribbe, 1983) as shown in Fig. 9, indicating no detectable strain is imposed on the orthoclase phase by the exsolution lamellae, which is expected given the dominance of the orthoclase phase in the RLS. The orthoclase phase shows a structure similar to the Himalaya Orthoclase from California (Prince et al., 1973), which has reached maximum ordering for a macroscopically monoclinic structure with ∼ 80 % of Al ordering into T1 sites (Smith and Brown, 1988, p. 44). The composition of the orthoclase phase from structure refinement is An0.2Ab12Or87Cn0.8, agreeing nicely with the EPMA analysis results. A second orthoclase domain, rotated ∼ 1.9∘ around an irrational axis relative to the main domain with significantly weaker reflections (∼ of the main domain), can be identified in Crystal 1 (green arrows in Fig. 7b), which could be from an orthoclase film sandwiched by an oligoclase spindle similar to that shown in Fig. 2b.
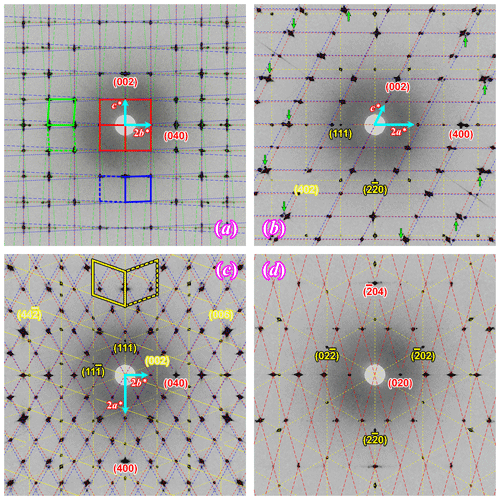
Figure 7Reconstructed sections of the reciprocal space from the SC-XRD data of Crystal 1. (a) 0kl image of oligoclase; (b) h0l image of orthoclase; (c) hk0 image of orthoclase; (d) image of magnetite film. The reciprocal lattice of orthoclase (dashed red lines), oligoclase (Albite twin law, dash-dotted green lines), albite (Pericline twin law, dash-dotted blue lines) and magnetite (Spinel twin law, dash-dotted yellow lines) are labeled with dash-dotted lines of different colors. The reciprocal unit cells are marked by the same color in (a) and (c) to clarify the lattices of different twin domains. Miller indices of a few characteristic reflections are also marked for orthoclase and one of the magnetite twin domains using the same color code. The albite reflections are not indexed as they are closely related to the adjacent orthoclase reflections.

Figure 8Off-centered sections of the reciprocal space showing some diffuse scattering features of the feldspar phases in Crystal 1. (a) h2l image of orthoclase showing diffuse streaking with that violates the C-centering lattice; (b) h8l image of orthoclase with reflection from the albite films (Pericline-twinned) showing obvious elongation along the [001]* direction; (c) hk4 image of orthoclase with a diffuse arc connecting reflections from albite and oligoclase. The reciprocal lattices are marked by the same colors as in Fig. 7.
The two oligoclase domains are related by the Albite twin law (marked with green lattices in Fig. 7), both showing sharp diffraction peaks with no obvious elongation or distortion along any direction, matching the micron-scaled twin lamellae observed with SEM (Fig. 2b). The refined structural composition of An28Ab72 matches the EPMA analysis. The oligoclase structure is very similar to those reported by Jin and Xu (2017) with split M sites and maximum Al–Si ordering (Table 4) even though the lattice parameters are slightly strained from typical oligoclase (Fig. 9). The thin films, on the other hand, are twinned following the Pericline law (marked with blue lattices in Fig. 7), consisting of nanoscaled (<100 nm) twin lamellae subparallel to the (001) plane, as indicated by the strongly elongated diffraction peaks along the [001]∗ direction (Fig. 8b), consistent with the transmission electron microscope (TEM) images of VSL295 from Evangelakakis et al. (1993). The composition of the thin films are shown to be almost pure albite from the structure refinement with no excess electron density on the M site relative to Na. The structure is intensely strained due to the interface with the orthoclase structure (Fig. 9). The b and c axes of the albite film are almost identical to the orthoclase matrix, suggesting perfect coherency at the interface. The 〈T–O〉 bond distances of the albite film shows a monoclinic topochemistry (Table 4) similar to the orthoclase matrix, with ∼ 75 % of the Al equally split between T1o and T1m sites. Arc-shaped streaks in the reconstructed sections of the reciprocal lattice, connecting the reflections from oligoclase and albite (arrow in Fig. 8c), are most likely from the transition between the oligoclase spindles and their albite film “tails” (arrows in Fig. 2).
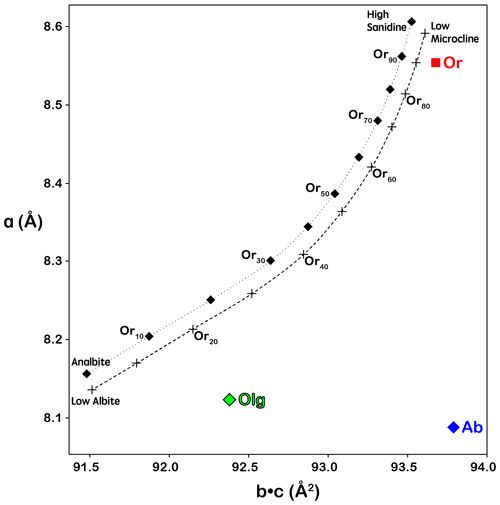
Figure 9Lattice dimension a plotted against b×c as proposed by W. H. Bernotat (Kroll and Ribbe, 1983) to show lattice strains on the feldspar structure. Unstrained alkali feldspars would have lattice parameters plotted in the narrow band between the dotted and dashed curves. Strained albite in a perthitic intergrowth would fall to the lower right side of the band, and strained orthoclase would plot to the upper left side of the band. The orthoclase structure plotted slightly to the right of the unstrained band probably due to the celsian (Ba) component in the composition.
The magnetite film (in Crystal 1) is Spinel-twinned, with a 180∘ rotation around the [111]* axis. The magnetite inclusion follows a specific crystallographic orientation relationship (COR) (Griffiths et al., 2016) with the host orthoclase: {111}Mt∥{100}Or and . No obvious elongation of the diffraction peaks of magnetite along the [111]* direction is observed despite the thin-film nature of the inclusion. This indicates the Spinel twin is not polysynthetic with multiple lamellae parallel to the (111) plane but is most likely comprised of only two continuous twin domains separated by one composition plane roughly in the middle of the magnetite film. The magnetite seed (in Crystal 2), although appearing randomly orientated, is also crystallographically constrained by the host orthoclase, with {111}Mt∥{110}Or and (see Fig. S3). No twinning is detected for the magnetite seed. Other CORs between the magnetite seeds and the orthoclase host probably also exist given the variety of crystal forms and apparent orientations (Fig. 6). No diffraction signal is detected for the particle of unidentified phase next to the magnetite seed (Fig. 6), suggesting it is probably not a single crystal but amorphous or polycrystalline material.
The lattice parameter of the magnetite film structure is obviously distorted from the cubic symmetry. Instead of the 90∘ angle between axes for the cubic crystal system, the magnetite film structure has ∘, which is a significant deviation from 90∘, much larger than the measurement error. This means the symmetry of the magnetite film is reduced from cubic ( to trigonal (). The structure is still reported in a face-centered pseudo-cubic unit cell for comparison with regular magnetite structures. Other than the distorted lattice, the magnetite film structure shows no obvious difference from a regular cubic magnetite structure. Even the extinction condition of the pseudo-d-glide plane is obeyed in the diffraction pattern (Fig. 7b, c). The deviation from cubic symmetry is most likely caused by surface strain from the extremely anisotropic crystal shape and the interface with feldspar. It has been reported that epitaxic magnetite films grown on BaTiO3 substrate would change their magnetic properties at low temperature in a way similar to the Verwey transition, resulting from the changes in the interfacial stress (Bohra et al., 2019). A similar effect may exist in the magnetite films in RLS, which would require further magnetic analyses to confirm. Relaxing the Fe occupancy in the refinement results in a composition of Fe2.85O4 with each Fe site only 95 % occupied. Twin fractions of 60 % and 40 % were calculated for the obverse and reverse twin domains in the refinement. The magnetite seed appears to have a regular cubic lattice and structure with space group symmetry of . The Fe occupancies for the octahedral and tetrahedral sites are also 95 % when relaxed in the refinement, the same as in the magnetite film structure. This apparent Fe deficiency in the structure refinement could be some artifact from the data reduction affected by peak overlapping between different phases, considering the lattice parameters are not obviously smaller than the standard magnetite structure (even though the data are collected at 100 K). It is also possible that Al and Ti substitution in the magnetite structure is the reason for the apparent vacancies in the structure, which would require nanoSIMS (nanoscale secondary ion mass spectrometry) or TEM analysis to confirm.
5.1 Exsolution textures of the RLS
The compositions of the different components in the RLS are plotted in Fig. 10. The solvus temperature of alkali feldspars is dependent on several factors including ordering state, interface coherency and, most importantly, An composition. The strain-free solvi of the ternary feldspar between 600 to 1000 ∘C under P=1 kbar (0.1 GPa) and P=8 kbar (0.8 GPa) are calculated using SOLVCAL (Wen and Nekvasil, 1994) with the parameters from Elkins and Grove (1990) and plotted in Fig. 11. Given that the pegmatites in the HRMC were emplaced during the Alice Spring Orogeny after the maximum pressure of 8–10 kbar (0.8–1 GPa) were registered, the RLS must have crystallized and exsolved under lower pressure. The solvus temperature near the composition of the RLS is mostly determined by the An component, with the solvus for 700 and 800 ∘C almost parallel to the Ab–Or join. The pressure does not have an obvious effect on the solvus temperature either; only the slope is slightly reduced at high pressure (Benisek et al., 2004). With an An# of ∼ 1.5, the RLS is estimated to have crystalized on or above the strain-free solvus at ∼ 750 ∘C.
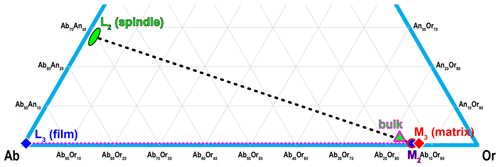
Figure 10The compositions of L2 (oligoclase spindle), L3 (albite film) and M3 (orthoclase matrix) are plotted on the ternary diagram, along with M2 (average of L3 and M3) and the bulk composition (average of L2, L3 and M3) of the RLS. The composition of Or96 reported by Liu et al. (2018) has to be incorrect as it places the RLS on the solvus far below the closure temperature for the exsolution process.
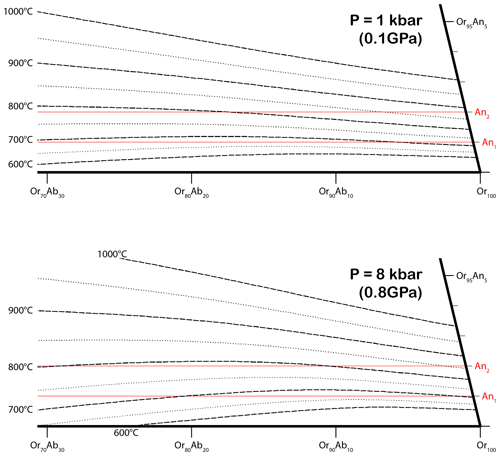
Figure 11Solvi of the ternary feldspar between 600 and 1000 ∘C with a 50 ∘C interval at P=1 kbar (0.1 GPa) and P=8 kbar (0.8 GPa), calculated with SOLVCAL (Wen and Nekvasil, 1994) using the parameters of Elkins and Grove (1990). The composition line with 1 mol % and 2 mol % An are marked with horizontal red lines. The An dimension is stretched for better separation between adjacent solvi. The more recent parameters of Benisek et al. (2010) are not used because they create a large discrepancy with the well-established binary solvus in the Ab–Or join.
All the exsolution lamellae (oligoclase spindles and albite films) in the RLS are pristine strain-controlled exsolution formed by coherent nucleation and growth, characterized by the unaltered isolated spindles and films with wedge terminations (Parsons and Brown, 1991). The “pull-aparts” in the oligoclase spindles (Fig. 2b), even though much larger in scale compared to those reported by Fitz Gerald et al. (2006), still have sharp pointy ends with no sign of “nano-tunnels” indicating fluid interaction. Assuming the coherent solvus is 50–100 ∘C below the strain-free solvus (Robin, 1974; Parsons and Brown, 1991), nucleation of the oligoclase spindles would initiate at ∼ 650 ∘C. The effect of strain on the shape of the coherent ternary solvus has not been systematically studied, but the coherent strain energy should increase with An# of the plagioclase due to the larger structural difference with the alkali feldspar, which may explain why only sodic plagioclase has been found to exsolve from alkali feldspars (Parsons and Brown, 1983; Brown and Parsons, 1988; Evangelakakis et al., 1993; Lee and Parsons, 1997, 2015; Abart et al., 2009a, b; Parsons and Lee, 2009; Parsons et al., 2009, 2013; Parsons and Fitz, Gerald, 2011; Tajčmanová et al., 2012). Pressure could also play a role in determining the plagioclase composition that nucleates from the orthoclase matrix even though its effect on the solvus temperature is small. As shown in Fig. 12, the strain-free tie lines passing through the bulk composition of the RLS at different temperatures are significantly shifted toward sodic compositions at high pressure. The spindle lamellae found in granulite (Evangelakakis et al., 1993; Abart et al., 2009a) do appear more sodic than those found in granite (Abart et al., 2009b), suggesting the coherent solvus may behave in a similar way. The oligoclase spindles are likely to be chemically zoned with decreasing An# from core to rim similar to the felsic granulites from the Bohemian Massif (Tajčmanová et al., 2012), resulting from falling temperature during spindle growth. Charactering the chemical variation within each individual spindle, however, would require techniques with higher spatial resolution than EPMA.
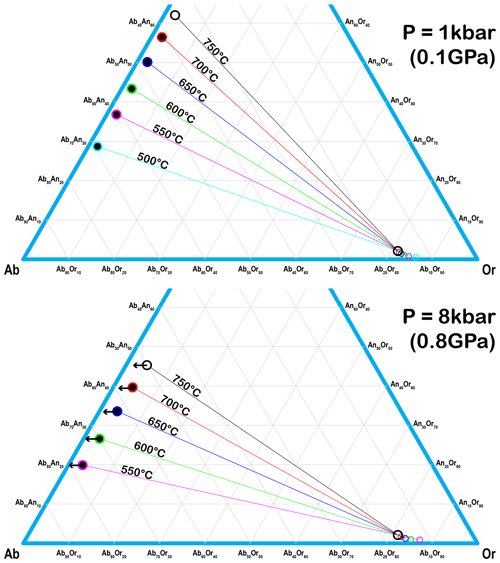
Figure 12Tie lines that pass through the bulk composition of the RLS at different temperatures under P=1 kbar (0.1 GPa) and P=8 kbar (0.8 GPa), calculated with SOLVCAL (Wen and Nekvasil, 1994) using the parameters of Elkins and Grove (1990). The plagioclase end of the tie lines shifts significantly towards sodic compositions at higher pressure.
The reason for the two-stage exsolution process, which is quite common in strain-controlled perthite (Brown and Parsons, 1983; Evangelakakis et al., 1993; Lee et al., 1995; Abart et al., 2009a, b; Tajčmanová et al., 2012), is self-explanatory from the composition plot in Fig. 10. Once the plagioclase nucleates, the An# cannot change due to the extremely slow interdiffusion between CaAl and NaSi (Kroll et al., 1993; Voll et al., 1994; Petrishcheva and Abart, 2012). The composition of the oligoclase spindle may re-equilibrate with the orthoclase matrix through Na–K interdiffusion as the temperature drops (Petrishcheva et al., 2014; Schäffer et al., 2014; Petrishcheva et al., 2020a) but only until they reach the An–Ab and Ab–Or join respectively. Upon further cooling, the almost Ca-free orthoclase matrix would enter the metastable region below the binary Ab–Or solvus, resulting in exsolution of albite films through nucleation and growth. Unlike those reported by Tajčmanová et al. (2012), the tail-like extensions on the oligoclase spindles (Fig. 2) have the same lengths and widths as the isolated film lamellae, suggesting they were formed simultaneously during cooling.
The albite films show a special monoclinic topochemistry (no Y ordering, only Z ordering; Thompson, 1969; Parsons and Brown, 1984) in the tetrahedral framework, indicating a two-step ordering scheme known only for K-rich feldspars. Albite with such an ordering pattern is only expected in ion-exchanged low sanidine or orthoclase (Smith and Brown, 1988, p. 145), which was shown to be extremely unstable in dry ion-exchange experiments (Horsky and Martin, 1977). The albite lamellae in Or-rich film perthite are often Pericline-twinned with composition planes (rhombic sections) close to the (001) cleavage, corresponding to high albite structure with a disordered framework (Parsons et al., 2015). With no detectable Ca in M2, the albite films in VSL295 (Evangelakakis et al., 1993) are estimated to have nucleated at 350–400 ∘C, far below the transition temperature between high and low albite. The “high-albite” structure at low temperature was attributed to inheriting the monoclinic framework of the orthoclase matrix. This explanation agrees with the known mechanism for the strain-controlled exsolution process in alkali feldspar, which initiates coherently with only K–Na interdiffusion but no change to the tetrahedral framework (Parsons and Brown, 1991; Petrishcheva and Abart, 2012; Petrishcheva et al., 2014; Schäffer et al., 2014; Petrishcheva et al., 2020a). The ordering pattern of the albite film in the RLS further supports this explanation as the two-step ordering (no Y ordering in the first step) is only known for the orthoclase structure. Contrary to the high exsolution temperature (due to An composition) proposed by Fitz Gerald et al. (2006), the monoclinic topochemistry of the albite film is actually evidence for low-temperature exsolution, which occurred after the ordering of the orthoclase framework below 500 ∘C (Brown and Parsons, 1989). The coherent strain from the orthoclase matrix must have stabilized the inherited framework in the albite films and strongly hindered (if not completely prohibited) further ordering after exsolution as the albite frameworks appear even less ordered than the orthoclase matrix (Table 4). In equilibrium with the albite films, the orthoclase matrix (M3) composition in the RLS (Or87) is slightly less potassic than the metamorphic perthites from Sri Lanka (> Or89) (Evangelakakis et al., 1993) likely due to a lower pressure at the closure temperature for exsolution.
5.2 Formation of the magnetite film
The hematite films are obviously not the original iron oxide inclusions but oxidized magnetite crystals instead. The thinner magnetite films (or the thinner parts) are apparently more prone to oxidation (Fig. 4a). Moonstones with only magnetite film inclusions showing no rainbow colors have also been reported from the mine (personal communication with the mine owner, 2021), which are not as attractive aesthetically to make it into the gemstone market. The triangular form of the film inclusions (Fig. 4c) is also typical for Spinel-twinned cubic crystals (Devouard et al., 1998; Daneu et al., 2007). The almost perfect lattice match between magnetite and orthoclase along the {111}Mt∥{100}Or interface (Fig. S4) could explain the uniform orientation and the anisotropy of the magnetite films that produce the “lattice” effect. The magnetite seeds suggest that the magnetite inclusions were formed at various orientations at the beginning, but only the ones that happened to follow the (100) plane of the orthoclase had a huge interfacial energy advantage which allows them to grow to extraordinary sizes. The same COR has been reported for magnetite inclusions in plagioclase (Ageeva et al., 2020) but presumably for a different reason as the needle shape in plagioclase suggests being the least favored interface, in contrast to RLS. The magnetite films were most likely oxidized during weathering of the host rock, considering that only a small portion was oxidized into hematite mostly around cracks in the crystal with generally undisturbed exsolution textures.
The magnetite inclusions in the RLS are formed around the same time as the exsolution of the oligoclase spindles at ∼ 650 ∘C. The possibility of Fe being introduced by external sources to the RLS can be excluded by the pristine strain-controlled albite lamellae. As it is known that the strain-loaded exsolution textures in alkali feldspars are very prone to hydrothermal or deuteric alteration even at low temperature (Brown, 1993; Parsons et al., 2015), the feldspar would quickly dissolve and reprecipitate around the lamellae boundaries under any influence of external Fe-rich (or Al-rich as proposed by Rosenqvist, 1951) fluid to release the strain. Therefore, the magnetite crystals must have exsolved from the iron dissolved in the feldspar lattice at crystallization, agreeing with the most recent TEM study of oriented magnetite micro-inclusions in plagioclase from oceanic gabbro, which shows direct evidence supporting precipitation from the Fe component of feldspar (Bian et al., 2021).
The valance state of Fe dissolved in the feldspar lattice is evidently critical for the formation of the magnetite films. Extensive work has been done on the oxidation state (Brown and Pritchard, 1968; Faye, 1969; Hafner et al., 1971; Hofmeister and Rossman, 1984; Petrov and Hafner, 1988; Tegner, 1997; Wilke et al., 2001; Sha et al., 2002; van Aken and Liebscher, 2002; Tegner and Cawthorn, 2010; Bourdelle et al., 2013; Nakada et al., 2019), as well as the partitioning of Fe in plagioclase feldspars (Sato, 1989; Phinney, 1992; Sugawara, 2000, 2001; Lundgaard and Tegner, 2004; Lac, 2009), mostly due to its significance in oxygen barometry in geologic systems. Partitioning of FeO and Fe2O3 between plagioclase and silicate melt was shown to be independent of both oxygen fugacity and plagioclase composition (Lundgaard and Tegner, 2004). Therefore, the average partition coefficient of Fe in plagioclase is dependent on the ratio as a function of oxygen fugacity. Similar studies in alkali feldspars, or K-feldspars in particular, are relatively limited. This is because Fe exists almost exclusively as Fe3+ in the tetrahedra framework replacing Al in K-feldspars (Michoulier and Gaite, 1972; Behrens et al., 1990; Ackermann et al., 2005). The Fe member of the feldspar group, ferrisanidine, which has been synthesized for decades (Faust, 1936; Wones and Appleman, 1963; Lebedeva et al., 2003; Taroev et al., 2008), was recently discovered in nature (Shchipalkina et al., 2019). However, most of the Fe-bearing alkali feldspars studied are K-rich sanidine that crystalized at high temperature, and the Fe concentrations of alkali feldspars from slow-cooled granite or pegmatite are significantly lower (Smith and Brown, 1988, pp. 310–327) probably due to the low Fe concentration in felsic magma. Little is known about how temperature, or the minor Ab and An component in K-feldspars, affects the behavior of Fe in the crystal lattice. For the extremely low Fe concentration in the RLS (<200 ppmw), it would be very difficult to analyze the ratio with any known method. Therefore, the following discussion will be based on previously published studies on Fe in feldspars.
The simplest possible equation for decomposing the Fe component of feldspar to produce magnetite inclusions is by proportional expulsion of Fe2+ in M sites (Fe) and Fe3+ in T sites (Fe) and creating M site vacancies ():
which can be separated into two simpler equations:
Equation (3) shows how excessive silica in the feldspar crystal is equivalent to M site vacancies as it can be written in the form of a feldspar formula as □1Si4O8 (Ribbe, 1983b). Note that Eq. (2) is the essence of Eq. (1) because K+, Al3+ or Si3+ is only balancing the charge. Therefore, M site vacancy () will be written in the form of silica (SiO2) for simplicity in the rest of this discussion unless the vacancy is directly involved in the reaction. Nonetheless, no evidence for Fe2+ in natural alkali feldspars has been reported (Behrens et al., 1990), meaning Eq. (1) alone cannot produce the magnetite inclusions in the RLS. The formation of magnetite films must involve the partial reduction of Fe3+ to Fe2+, assuming that Fe only exists as Fe3+ in the tetrahedra sites:
The K atoms could diffuse out of the feldspar crystal and evaporate similar to Na2O as suggested by Behrens et al. (1990). Moreover, a lot more SiO2 is produced in Eq. (4) compared to Eq. (2) for each formula of Fe3O4, which might exsolve from the feldspar and explain the particle of unknown phase in the RLS accompanying the magnetite seeds (Fig. 6).
It is very difficult to analyze exactly how much Fe has exsolved from the feldspar lattice in the RLS. Nonetheless, no correlation between the Fe concentration and the presence of visible inclusions in the laser spots of the LA-ICP-MS analysis is observed, suggesting that the majority of Fe in the RLS is still dissolved in the feldspar lattice. The magnetic analysis by Nakada et al. (2019) also shows that the exsolved magnetite inclusions only account for a small fraction of iron in the plagioclase sample. Equation (4) also conceivably provides a driving force for the expulsion of Fe from the feldspar lattice even at such low concentrations, given that Fe2+ is much less soluble than Fe3+, especially in alkali feldspars. This explains why no correlation among the bulk Fe concentration, cooling rates and the prominence of iron oxide inclusions has been observed in natural feldspars (Smith and Brown, 1988, p. 637) as the solubility of Fe in feldspars is much more sensitive to oxygen fugacity than temperature. Behrens et al. (1990) have demonstrated that the oxidation state of Fe in plagioclase crystals can be altered relatively easily under solid state at high temperature (1250 ∘C) through internal redox reactions, indicating that the oxidizing/reducing agents (mainly in the form of M site vacancies) can diffuse relatively fast into or out of the feldspar crystals. The lamellae boundaries in the RLS would also facilitate the diffusion of the oxidizing/reducing agent by creating a network of express pathways (Abart et al., 2009a, b; Tajčmanová et al., 2012).
The magnetite films in the RLS are large and well-separated from each other, indicating Eq. (4) did not result in the immediate precipitation of magnetite, which would create clouds of tiny precipitates as described by Behrens et al. (1990). The reduced Fe atoms need to migrate on the millimeter scale before precipitating on a magnetite seed or film nucleus. Unfortunately, little is known about the diffusion coefficient or mechanism of Fe in feldspars other than that Fe2+ diffuses more than 3 orders of magnitudes faster than Fe3+ at 1200 ∘C in labradorite (Behrens et al., 1990). Nonetheless, to reduce Fe in the feldspar without immediate precipitation of any extra solid phase, some of the Fe2+ has to come out of the tetrahedra sites:
where the interstitial Fe can also enter the M site vacancies:
It should be noted that Eq. (5) is not reversible at high oxygen fugacity because oxidizing interstitial Fe (or Fe in the M site) and transforming it to the tetrahedra site are very unlikely (Behrens et al., 1990). The remaining Fe2+ in the tetrahedra sites can also come out by precipitating/evaporating K2O:
The combination of Eqs. (5), (6), (7) and (2) is Eq. (4). Note that Eqs. (5), (6) and (7) are only intermediate steps that allow the migration of Fe inside the feldspar crystal before precipitation. Therefore, the amount of Fe2+ in the feldspar lattice could be far below the detection limit of any analytical method. Equation (4) produces a large amount of M site vacancies (excessive silica) which not only facilitate the reduction of Fe (Eqs. 5 + 6) but also promote the fast diffusion of Fe2+ in feldspar (Eq. 6) (Behrens et al., 1990). Once the magnetite crystals are precipitated, they can only keep growing but cannot move or be dissolved again. Therefore, the initial number of nuclei, which is mostly sensitive to the cooling rate, is critical in determining the final appearance of the crystal, as shown in the simulation by Abart et al. (2009b).
The equations shown above may also be applied to plagioclase feldspars simply by changing K to Na, with the main difference being that detectable amounts of Fe2+ can exist in the plagioclase lattice, mostly in the tetrahedra sites with an end-member formula of CaFeSi3O8 (Sclar and Kastelic, 1979; Behrens et al., 1990; Sugawara, 2000, 2001). This means Eqs. (5) and (6) may explain the absence of clouding in some labradorites after heating experiments at low oxygen fugacity by Behrens et al. (1990). Bian et al. (2021) proposed equations that involve pyroxene for precipitating magnetite from plagioclase, which also indicate low oxygen fugacity as the driving force, agreeing with the observation by Nakada et al. (2019) that the clouded plagioclase samples are more reduced than the clear ones. However, Bian et al. (2021) did not consider □1Si4O8 as a phase component while theorizing their reaction; thus a significant amount of Fe2+ in M site (Fe) is required to balance the equation, which cannot be the case for alkali feldspars such as RLS. It should also be noted that ferrosilite pyroxene is simply the product of the incomplete decomposition of Fe in feldspar, which can further transform to magnetite by reacting with Fe3+ at the T site (Fe):
meaning it is not a necessary byproduct for precipitating magnetite from feldspar.
The hematite inclusions in red-clouded or aventurine feldspars are harder to explain by direct exsolution without an obvious force for the expulsion of Fe3+, which is one of the reasons why metasomatism is the preferred mechanism. The RLS has provided an alternative mechanism for the hematite inclusions in clear pristine feldspar crystals, which is through oxidation of previously exsolved magnetite films or flakes. This was not considered before mainly because the cubic magnetite structure in flaky crystal form is counterintuitive. Some hematite inclusions with a similar appearance as in the RLS have been found in Tanzania (Koivula and Tannous, 2003) and North Carolina, USA (Challener et al., 2017). The morphology of the iron oxide inclusion seems to be correlated with the composition and structure of the host feldspar, with acicular inclusions found only in intermediate to calcic plagioclase (Armbrustmacher and Banks, 1974; Nienaber-Roberts, 1986; Sobolev, 1990; Wenk et al., 2011; Ageeva et al., 2020; Jin et al., 2021; Bian et al., 2021) and flaky inclusions mostly in sodic plagioclase and alkali feldspar (Boone, 1969; Copley and Gay, 1979; Challener et al., 2017; Liu et al., 2018).
The strain-controlled exsolution textures in the RLS are very unusual, especially for its composition and scale. Elongated belt-shaped pristine lamellae that are several micrometers thick have not been reported before. The “pull-aparts” in the RLS accommodating the lattice strain on the interfaces are not evenly distributed in every oligoclase spindle, and they only appear in (010) sections but not in (001) sections. The wide variety of different sizes and shapes of the film perthite, from nano-platelets to thin films to spindles, is likely due to the combined effect of chemical composition, pressure and cooling rate. Several aspects of the strain-controlled exsolution textures in alkali feldspars may be explored in future studies, including the effect of pressure on the composition of the plagioclase lamellae, as well as the chemical zoning in the plagioclase lamellae in alkali feldspars as a function of cooling rate. The structural transition from oligoclase spindles to albite films may also reveal some details about the nucleation and growth process.
SC-XRD has been shown to be a powerful tool for studying the submicron inclusions and exsolution lamellae in minerals and is complementary to electron microscopy. It is especially well-suited for strain quantification and structure analysis in strain-controlled exsolution textures (Jayaraman, 1959; MacKenzie and Smith, 1962; Ribbe, 1979). Unlike intergrowths formed by replacement (Balić-Žunić et al., 2013), different lamellae of the same phase in diffusion-formed intergrowth have exactly the same orientation and therefore produce the same diffraction pattern. The strained structure of the albite lamellae in film perthite is refined for the first time, revealing a monoclinic topochemistry inheriting the framework from the orthoclase host. This is further evidence supporting the framework distortion or collapsing as being the main driving force for the Al–Si ordering in plagioclase feldspar (Jin et al., 2019). It also demonstrates that the ordering state may provide additional constraints on the nucleation temperature of the exsolution lamellae. Some previously studied film perthite (such as the Shap granite) should be revisited with SC-XRD to see if there are any structure variations in the albite films due to different composition and cooling rate.
The unusual relation between the magnetite films and the exsolution textures in the RLS reveals a new forming mechanism for the enigmatic Fe-bearing inclusions commonly found in feldspar minerals. Feldspar samples with different forms of iron oxide inclusions and exsolution textures from other localities, such as the popular peach moonstone or black moonstone on the gem market, may provide additional clues for a more complete picture. The exact atomic configuration at the magnetite-film surface may provide more details about the nucleation and growth process in included feldspars. The detailed chemical composition profiles across these boundaries can be analyzed using atom probe tomography (APT) or analytical TEM, and the trace element concentrations in each individual phase can be analyzed with nanoSIMS, which may help us to understand the chemical diffusion process during the nucleation and growth.
The detailed crystallographic information of the refined structures, including the structure factors integrated from the raw diffraction data, is provided in the CIF in the Supplement.
The supplement related to this article is available online at: https://doi.org/10.5194/ejm-34-183-2022-supplement.
SJ and ACP conceptualized the project and designed the experiments. SJ and ZS carried out the experiments. SJ analyzed the data and interpreted the results. SJ prepared the manuscript with contributions from all co-authors.
The contact author has declared that neither they nor their co-authors have any competing interests.
Publisher’s note: Copernicus Publications remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
This research is supported by the Richard T. Liddicoat Postdoctoral Research Associate Fellowship program at GIA. The authors thank Nicolas M. Roberts for acquiring the rainbow lattice sunstone sample from Australia; Karen V. Smit and Elina Myagkaya for preliminary SEM work; Chi Ma for assisting with the EPMA analyses and SEM imaging of the sample; and Milan Gembicky for helping design the strategy and collect the SC-XRD data. We also thank Rainer Abart and two anonymous reviewers for many constructive comments, and associate editor Giuseppe Cruciani for handling the manuscript.
This paper was edited by Giuseppe Cruciani and reviewed by two anonymous referees.
Abart, R., Petrishcheva, E., Wirth, R., and Rhede, D.: Exsolution by spinodal decomposition II: Perthite formation during slow cooling of anatexites from Ngoronghoro, Tanzania, Am. J. Sci., 309, 450–475, https://doi.org/10.2475/06.2009.02, 2009a.
Abart, R., Petrishcheva, E., Käßner, S., and Milke, R.: Perthite microstructure in magmatic alkali feldspar with oscillatory zoning; Weinsberg Granite, Upper Austria, Mineral. Petrol., 97, 251, https://doi.org/10.1007/s00710-009-0090-1, 2009b.
Ackermann, S., Kunz, M., Armbruster, T., Schefer, J., and Hanni, H.: Cation distribution in a Fe-bearing K-feldspar from Itrongay, Madagascar. A combined neutron- and X-ray single crystal diffraction study, Schweizaerische Mineral. Petrogr. Mitteilungen, 84, 345–354, 2005.
Ageeva, O., Bian, G., Habler, G., Pertsev, A., and Abart, R.: Crystallographic and shape orientations of magnetite micro-inclusions in plagioclase, Contrib. Mineral. Petr., 175, 95, https://doi.org/10.1007/s00410-020-01735-8, 2020.
Andersen, O.: On aventurine feldspar, Am. J. Sci., s4-40, 351–399, https://doi.org/10.2475/ajs.s4-40.238.351, 1915.
Armbrustmacher, T. J. and Banks, N. G.: Clouded plagioclase in metadolerite dikes, Southeastern Bighorn Mountains, Wyoming, Am. Mineral., 59, 656–665, 1974.
Balić-Žunić, T., Piazolo, S., Katerinopoulou, A., and Schmith, J. H.: Full analysis of feldspar texture and crystal structure by combining X-ray and electron techniques, Am. Mineral., 98, 41–52, https://doi.org/10.2138/am.2013.4124, 2013.
Behrens, H., Johannes, W., and Schmalzried, H.: On the Mechanisms of Cation Diffusion Processes in Ternary Feldspars, Phys. Chem. Miner., 17, 62–78, https://doi.org/10.1007/BF00209227, 1990.
Benisek, A., Kroll, H., and Cemič, L.: New developments in two-feldspar thermometry, Am. Mineral., 89, 1496–1504, https://doi.org/10.2138/am-2004-1018, 2004.
Benisek, A., Dachs, E., and Kroll, H.: A ternary feldspar-mixing model based on calorimetric data: development and application, Contrib. Mineral. Petrol., 160, 327–337, https://doi.org/10.1007/s00410-009-0480-8, 2010.
Bian, G., Ageeva, O., Rečnik, A., Habler, G., and Abart, R.: Formation pathways of oriented magnetite micro-inclusions in plagioclase from oceanic gabbro, Contrib. Mineral. Petrol., 176, 104, https://doi.org/10.1007/s00410-021-01864-8, 2021.
Bohra, M., Agarwal, N., and Singh, V.: A Short Review on Verwey Transition in Nanostructured Fe3O4 Materials, J. Nanomater., 2019, e8457383, https://doi.org/10.1155/2019/8457383, 2019.
Boone, G. M.: Origin of clouded red feldspars: petrologic contrasts in a granitic porphyry intrusion, Am. J. Sci., 267, 633–668, https://doi.org/10.2475/ajs.267.6.633, 1969.
Bourdelle, F., Benzerara, K., Beyssac, O., Cosmidis, J., Neuville, D. R., Brown, G. E., and Paineau, E.: Quantification of the ferric/ferrous iron ratio in silicates by scanning transmission X-ray microscopy at the Fe L2,3 edges, Contrib. Mineral. Petr., 166, 423–434, https://doi.org/10.1007/s00410-013-0883-4, 2013.
Brown, F. F. and Pritchard, A. M.: The Mössbauer Spectrum of Iron Orthoclase, Earth Planet. Sc. Lett., 5, 259–260, https://doi.org/10.1016/S0012-821X(68)80049-4, 1968.
Brown, W. L.: Stored elastic strain energy: the driving force for low-temperature reactivity and alteration of alkali feldspar, in: Defects and processes in solid state: Geoscience applications, edited by: Boland, J. N. and Fitz Gerald, J. D., Elsevier, Amsterdam, 267–290, https://doi.org/10.1016/0009-2541(95)90027-6, 1993.
Brown, W. L. and Parsons, I.: Nucleation on perthite-perthite boundaries and exsolution mechanisms in alkali feldspars, Phys. Chem. Miner., 10, 55–61, https://doi.org/10.1007/BF00309585, 1983.
Brown, W. L. and Parsons, I.: Zoned ternary feldspars in the Klokken intrusion: exsolution microtextures and mechanisms, Contrib. Mineral. Petr., 98, 444–454, https://doi.org/10.1007/BF00372364, 1988.
Brown, W. L. and Parsons, I.: Alkali feldspars: ordering rates, phase transformations and behaviour diagrams for igneous rocks, Mineral. Mag., 53, 25–42, https://doi.org/10.1180/minmag.1989.053.369.03, 1989.
Buick, I. S., Miller, J. A., Williams, I. S., and Cartwright, I.: Ordovician high-grade metamorphism of a newly recognised late Neoproterozoic terrane in the northern Harts Range, central Australia, J. Metamorph. Geol., 19, 373–394, https://doi.org/10.1046/j.0263-4929.2001.00316.x, 2001.
Buick, I. S., Hand, M., Williams, I. S., Mawby, J., Miller, J. A., and Nicoll, R. S.: Detrital zircon provenance constraints on the evolution of the Harts Range Metamorphic Complex (central Australia): links to the Centralian Superbasin, J. Geol. Soc., 162, 777–787, https://doi.org/10.1144/0016-764904-044, 2005.
Buick, I. S., Storkey, A., and Williams, I. S.: Timing relationships between pegmatite emplacement, metamorphism and deformation during the intra-plate Alice Springs Orogeny, central Australia, J. Metamorph. Geol., 26, 915–936, https://doi.org/10.1111/j.1525-1314.2008.00794.x, 2008.
Challener, S., Renfro, N., and Sun, Z.: New Phenomenal Feldspar from North Carolina with Iridescent Inclusions, Gems Gemol., 53, p. 467, 2017.
Copley, P. A. and Gay, P.: Crystallographic studies of some Norwegian aventurinised feldspars by optical, X-ray, and electron optical methods, Nor. Geol. Tidsskr., 59, 229–237, 1979.
Daneu, N., Rečnik, A., Yamazaki, T., and Dolenec, T.: Structure and chemistry of (111) twin boundaries in MgAl2O4 spinel crystals from Mogok, Phys. Chem. Miner., 34, 233–247, https://doi.org/10.1007/s00269-007-0142-1, 2007.
Devouard, B., Posfai, M., Hua, X., Bazylinski, D. A., Frankel, R. B., and Buseck, P. R.: Magnetite from magnetotactic bacteria: Size distributions and twinning, Am. Mineral., 83, 1387–1398, https://doi.org/10.2138/am-1998-11-1228, 1998.
Elkins, L. T. and Grove, T. L.: Ternary feldspar experiments and thermodynamic models, Am. Mineral., 75, 544–559, 1990.
Evangelakakis, C., Kroll, H., Voll, G., Wenk, H.-R., Meisheng, H., and Köpcke, J.: Low-temperature coherent exsolution in alkali feldspars from high-grade metamorphic rocks of Sri Lanka, Contrib. Mineral. Petr., 114, 519–532, https://doi.org/10.1007/BF00321756, 1993.
Faust, G. T.: The Fusion Relations of Iron-Orthoclase, Am. Mineral., 21, 735–763, 1936.
Faye, G. H.: The optical absorption spectrum of tetrahedrally bonded Fe3+ in orthoclase, Can. Mineral., 10, 112–117, 1969.
Fitz Gerald, J. D., Parsons, I., and Cayzer, N.: Nanotunnels and pull-aparts: Defects of exsolution lamellae in alkali feldspars, Am. Mineral., 91, 772–783, https://doi.org/10.2138/am.2006.2029, 2006.
Griffiths, T. A., Habler, G., and Abart, R.: Crystallographic orientation relationships in host–inclusion systems: New insights from large EBSD data sets, Am. Mineral., 101, 690–705, https://doi.org/10.2138/am-2016-5442, 2016.
Hafner, S. S., Virgo, D., and Warburton, D.: Oxidation state of iron in plagioclase from lunar basalts, Earth Planet. Sc. Lett., 12, 159–166, https://doi.org/10.1016/0012-821X(71)90072-0, 1971.
Hand, M., Mawby, J., Kinny, P., and Foden, J.: U–Pb ages from the Harts Range, central Australia: evidence for early Ordovician extension and constraints on Carboniferous metamorphism, J. Geol. Soc., 156, 715–730, https://doi.org/10.1144/gsjgs.156.4.0715, 1999.
Hofmeister, A. M. and Rossman, G. R.: COLOR in FELDSPARS, in: Feldspar Mineralogy, edited by: Ribbe, P. H., Mineralogical Society of American, Washington, D.C., 271–280, https://doi.org/10.1515/9781501508547, 1983.
Hofmeister, A. M. and Rossman, G. R.: Determination of Fe3+ and Fe2+ concentrations in feldspar by optical-absorption and electron-paramagnetic-res spectroscopy, Phys. Chem. Miner., 11, 213–224, https://doi.org/10.1007/Bf00308136, 1984.
Horsky, S. J. and Martin, R. F.: The anomalous ion-exchange behavior of “ordered” orthoclase, Am. Mineral., 62, 1191–1199, 1977.
Jayaraman, A.: X-ray study of the structure of moonstones, P. Indian Acad. Sci. A, 50, 349–359, https://doi.org/10.1007/BF03048927, 1959.
Jin, S. and Xu, H.: Investigations of the phase relations among e1, e2 and structures of Na-rich plagioclase: a single-crystal X-ray diffraction study, Acta Crystallogr. B, 73, 992–1006, https://doi.org/10.1107/S2052520617010976, 2017.
Jin, S., Xu, H., Wang, X., Zhang, D., Jacobs, R., and Morgan, D.: The incommensurately modulated structures of volcanic plagioclase: displacement, ordering and phase transition, Acta Crystallogr. B, 75, 643–656, https://doi.org/10.1107/S2052520619006243, 2019.
Jin, S., Xu, H., and Lee, S.: Revisiting the Bøggild Intergrowth in Iridescent Labradorite Feldspars: Ordering, Kinetics, and Phase Equilibria, Minerals, 11, 727, https://doi.org/10.3390/min11070727, 2021.
Joklik, G. F.: The geology and mica-fields of the Harts Range, central Australia, Govt. Printing Office, Canberra, 226 pp., https://ecat.ga.gov.au/geonetwork/srv/api/records/a05f7892-7fe0-7506-e044-00144fdd4fa6 (last access: 23 March 2022), 1955a.
Joklik, G. F.: The mica-bearing pegmatites of the Harts range, central Australia, Econ. Geol., 50, 625–649, https://doi.org/10.2113/gsecongeo.50.6.625, 1955b.
Koivula, J. I. and Gunter, M. E.: Gem News: A beautiful new form of orthoclase, Gems Gemol., 25, 47, 1989.
Koivula, J. I. and Tannous, M.: Star sunstone from Tanzania, Gems Gemol., 39, 235–236, 2003.
Kroll, H. and Ribbe, P. H.: Lattice parameters, composition and Al, Si order in alkali feldspars, in: Feldspar Mineralogy, edited by: Ribbe, P. H., Mineralogical Society of American, Washington, D.C., 57–100, https://doi.org/10.1515/9781501508547, 1983.
Kroll, H., Evangelakakis, C., and Voll, G.: Two-feldspar geothermometry: a review and revision for slowly cooled rocks, Contrib. Mineral. Petr., 114, 510–518, https://doi.org/10.1007/BF00321755, 1993.
Lac, D.: Using the Oxidation State of Iron in Plagioclase to Evaluate Magma Oxygen Fugacity: A micro-XANES Study, Masters Thesis, University of Massachusetts-Amherst, Amherst, MA, USA, 80 pp., https://scholarworks.umass.edu/theses/339/ (last access: 23 March 2022), 2009.
Lebedeva, Y. S., Pushcharovsky, D. Y., Pasero, M., Merlino, S., Kashaev, A. A., Taroev, V. K., Goettlicher, J., Kroll, H., Pentinghaus, H., Suvorova, L. F., Wulf-Bernodat, H., and Lashkevich, V. V.: Synthesis and crystal structure of low ferrialuminosilicate sanidine, Crystallogr. Rep.+, 48, 919–924, https://doi.org/10.1134/1.1627432, 2003.
Lee, M. R. and Parsons, I.: Dislocation formation and albitization in alkali feldspars from the Shap Granite, Am. Mineral., 82, 557–570, https://doi.org/10.2138/am-1997-5-616, 1997.
Lee, M. R. and Parsons, I.: Diffusion-controlled and replacement microtextures in alkali feldspars from two pegmatites: Perth, Ontario and Keystone, South Dakota, Mineral. Mag., 79, 1711–1735, https://doi.org/10.1180/minmag.2015.079.7.21, 2015.
Lee, M. R., Waldron, K. A., and Parsons, I.: Exsolution and alteration microtextures in alkali feldspar phenocrysts from the Shap granite, Mineral. Mag., 59, 63–78, https://doi.org/10.1180/minmag.1995.59.394.06, 1995.
Liu, J., Shen, A. H., Zhang, Z., Wang, C., and Shao, T.: Revisiting Rainbow Lattice Sunstone from the Harts Range, Australia, J. Gemmol., 36, 44–52, 2018.
Lundgaard, K. L. and Tegner, C.: Partitioning of ferric and ferrous iron between plagioclase and silicate melt, Contrib. Mineral. Petr., 147, 470–483, https://doi.org/10.1007/s00410-004-0568-0, 2004.
MacKenzie, W. S. and Smith, J. V.: Single crystal X-ray studies of crypto-and micro-perthites, Nor. Geol. Tidsskr., 42, 72–103, 1962.
Mawby, J., Hand, M., and Foden, J.: Sm–Nd evidence for high-grade Ordovician metamorphism in the Arunta Block, central Australia, J. Metamorph. Geol., 17, 653–668, https://doi.org/10.1046/j.1525-1314.1999.00224.x, 1999.
McConnell, J. D. C.: Electron-optical study of the fine structure of a schiller labradorite, in: The Feldspars, edited by: MacKenzie, W. S. and Zussman, J., Manchester University Press, New York, 478–490, ISBN 978-0-7190-0544-2, 1974.
Michoulier, J. and Gaite, J. M.: Site Assignment of Fe3+ in Low Symmetry Crystals. Application to NaAlSi3O8, J. Chem. Phys., 56, 5205–5213, https://doi.org/10.1063/1.1677022, 1972.
Momma, K. and Izumi, F.: VESTA 3 for three-dimensional visualization of crystal, volumetric and morphology data, J. Appl. Crystallogr., 44, 1272–1276, https://doi.org/10.1107/S0021889811038970, 2011.
Nakada, R., Sato, M., Ushioda, M., Tamura, Y., and Yamamoto, S.: Variation of Iron Species in Plagioclase Crystals by X-ray Absorption Fine Structure Analysis, Geochem. Geophy. Geosy., 20, 5319–5333, https://doi.org/10.1029/2018gc008131, 2019.
Nienaber-Roberts, C. J.: A note on the needlelike inclusions in plagioclase from the Bushveld Complex, Ann. Van Geol. Opname Departement Van Miner.-En Energiesake, 20, 55–58, 1986.
Parsons, I. and Brown, W. L.: A TEM and microprobe study of a two-perthite alkali gabbro: Implications for the ternary feldspar system, Contrib. Mineral. Petr., 82, 1–12, https://doi.org/10.1007/BF00371170, 1983.
Parsons, I. and Brown, W. L.: Feldspars and the Thermal History of Igneous Rocks, in: Feldspars and Feldspathoids: Structures, Properties and Occurrences, edited by: Brown, W. L., Springer Netherlands, Dordrecht, 317–371, https://doi.org/10.1007/978-94-015-6929-3_9, 1984.
Parsons, I. and Brown, W. L.: Mechanisms and Kinetics of Exsolution – Structural Control of Diffusion and Phase Behavior in Alkali Feldspars, in: Diffusion, Atomic Ordering, and Mass Transport, Springer-Verlag, New York, 304–344, https://doi.org/10.1007/978-1-4613-9019-0, 1991.
Parsons, I. and Fitz Gerald, J. D.: Coarsening kinetics of coexisting peristerite and film microperthite over 104 to 105 years, Am. Mineral., 96, 1575–1584, https://doi.org/10.2138/am.2011.3770, 2011.
Parsons, I. and Lee, M. R.: Mutual replacement reactions in alkali feldspars I: microtextures and mechanisms, Contrib. Mineral. Petrol., 157, 641–661, https://doi.org/10.1007/s00410-008-0355-4, 2009.
Parsons, I., Magee, C. W., Allen, C. M., Shelley, J. M. G., and Lee, M. R.: Mutual replacement reactions in alkali feldspars II: trace element partitioning and geothermometry, Contrib. Mineral. Petr., 157, 663, https://doi.org/10.1007/s00410-008-0358-1, 2009.
Parsons, I., Fitz Gerald, J. D., Heizler, M. T., Heizler, L. L., Ivanic, T., and Lee, M. R.: Eight-phase alkali feldspars: low-temperature cryptoperthite, peristerite and multiple replacement reactions in the Klokken intrusion, Contrib. Mineral. Petr., 165, 931–960, https://doi.org/10.1007/s00410-012-0842-5, 2013.
Parsons, I., Fitz Gerald, J. D., and Lee, M. R.: Routine characterization and interpretation of complex alkali feldspar intergrowths, Am. Mineral., 100, 1277–1303, https://doi.org/10.2138/am-2015-5094, 2015.
Petříček, V., Dušek, M., and Palatinus, L.: Crystallographic Computing System JANA2006: General features, Z. Krist.-Cryst. Mater., 229, 345–352, https://doi.org/10.1515/zkri-2014-1737, 2014.
Petrishcheva, E. and Abart, R.: Exsolution by spinodal decomposition I: Evolution equation for binary mineral solutions with anisotropic interfacial energy, Am. J. Sci., 309, 431–449, https://doi.org/10.2475/06.2009.01, 2009.
Petrishcheva, E. and Abart, R.: Exsolution by spinodal decomposition in multicomponent mineral solutions, Acta Mater., 60, 5481–5493, https://doi.org/10.1016/j.actamat.2012.07.006, 2012.
Petrishcheva, E., Abart, R., Schäffer, A.-K., Habler, G., and Rhede, D.: Sodium-potassium interdiffusion in potassium-rich alkali feldspar I: Full diffusivity tensor at 850 ∘C, Am. J. Sci., 314, 1284–1299, https://doi.org/10.2475/09.2014.02, 2014.
Petrishcheva, E., Tiede, L., Heuser, D., Hutter, H., Giester, G., and Abart, R.: Multicomponent diffusion in ionic crystals: theoretical model and application to combined tracer- and interdiffusion in alkali feldspar, Phys. Chem. Miner., 47, 35, https://doi.org/10.1007/s00269-020-01103-9, 2020a.
Petrishcheva, E., Tiede, L., Schweinar, K., Habler, G., Li, C., Gault, B., and Abart, R.: Spinodal decomposition in alkali feldspar studied by atom probe tomography, Phys. Chem. Miner., 47, 30, https://doi.org/10.1007/s00269-020-01097-4, 2020b.
Petrov, I. and Hafner, S. S.: Location of trace Fe3+ ions in sanidine, KAlSi3O8, Am. Mineral., 73, 97–104, 1988.
Phinney, W. C.: Partition coefficients for iron between plagioclase and basalt as a function of oxygen fugacity: Implications for Archean and lunar anorthosites, Geochim. Cosmochim. Ac., 56, 1885–1895, https://doi.org/10.1016/0016-7037(92)90318-D, 1992.
Prince, E., Donnay, G., and Martin, R. F.: Neutron diffraction refinement of an ordered orthoclase structure, Am. Mineral., 58, 500–507, 1973.
Ribbe, P. H.: The structure of a strained intermediate microcline in cryptoperthitic association with twinned plagioclase, Am. Mineral., 64, 402–408, 1979.
Ribbe, P. H.: Exsolution textures in ternary and plagioclase feldspars; interference colors, in: Feldspar Mineralogy, edited by: Ribbe, P. H., Mineralogical Society of American, Washington, D.C., 241–270, https://doi.org/10.1515/9781501508547, 1983a.
Ribbe, P. H.: The chemistry, structure and nomenclature of feldspars, in: Feldspar Mineralogy, edited by: Ribbe, P. H., Mineralogical Society of American, Washington, D.C., 1–19, https://doi.org/10.1515/9781501508547, 1983b.
Robin, P.-Y. F.: Stress and strain in cryptoperthite lamellae and the coherent solvus of alkali feldspars, Am. Mineral., 59, 1299–1318, 1974.
Rochow, K.: An Investigation of the Harts Range and Plenty River Mica Mines, Bureau of Mineral Resources, Geology and Geophysics, https://ecat.ga.gov.au/geonetwork/srv/api/records/a05f7892-97ce-7506-e044-00144fdd4fa6 (last access: 23 March 2022), 1962.
Rosenqvist, I. T.: Investigations in the crystal chemistry of silicates III. The Relation Haematite-Microcline, Nor. Geol. Tidsskr., 29, 65–76, 1951.
Sato, H.: Mg-Fe Partitioning between Plagioclase and Liquid in Basalts of Hole 504B, ODP Leg 111: A Study of Melting at 1 ATM, Proc. Ocean Drill. Program Sci. Results, 111, 17–26, https://doi.org/10.2973/odp.proc.sr.111.1989, 1989.
Schäffer, A.-K., Petrishcheva, E., Habler, G., Abart, R., Rhede, D., and Giester, G.: Sodium-potassium interdiffusion in potassium-rich alkali feldspar II: Composition- and temperature-dependence obtained from cation exchange experiments, Am. J. Sci., 314, 1300–1318, https://doi.org/10.2475/09.2014.03, 2014.
Sclar, C. B. and Kastelic, R. L.: Iron in Anorthite: an Experimental Study, in: Lunar and planetary science X, 1087–1089, https://www.lpi.usra.edu/meetings/lpsc1979/ (last access: 23 March 2022), 1979.
Scrimgeour, I.: Chapter 29: IRINDINA PROVINCE, in: Geology and mineral resources of the Northern Territory, edited by: Ahmad, M. and Munson, T., ISBN 978-07-2457-257-1, 2013.
Sha, Y., Zhang, P., Wang, G., Zhang, X., and Wang, X.: Determination of the chemical states of iron in plagioclase by external beam ionoluminescence, Nucl. Instrum. Meth. B, 189, 408–411, https://doi.org/10.1016/S0168-583X(01)01100-4, 2002.
Shchipalkina, N. V., Pekov, I. V., Britvin, S. N., Koshlyakova, N. N., Vigasina, M. F., and Sidorov, E. G.: A New Mineral Ferrisanidine, K[Fe3+Si3O8], the First Natural Feldspar with Species-Defining Iron, Minerals, 9, 770, https://doi.org/10.3390/min9120770, 2019.
Smith, J. V. and Brown, W. L.: Feldspar Minerals, Springer-Verlag, Berlin, Heidelberg, 828 pp., 1988.
Sobolev, P. O.: Orientation of acicular iron-ore mineral inclusions in plagioclase, Int. Geol. Rev., 32, 616–628, https://doi.org/10.1080/00206819009465804, 1990.
Sugawara, T.: Thermodynamic analysis of Fe and Mg partitioning between plagioclase and silicate liquid, Contrib. Mineral. Petr., 138, 101–113, https://doi.org/10.1007/s004100050011, 2000.
Sugawara, T.: Ferric iron partitioning between plagioclase and silicate liquid: thermodynamics and petrological applications, Contrib. Mineral. Petr., 141, 659–686, https://doi.org/10.1007/s004100100267, 2001.
Tajčmanová, L., Abart, R., Wirth, R., Habler, G., and Rhede, D.: Intracrystalline microstructures in alkali feldspars from fluid-deficient felsic granulites: a mineral chemical and TEM study, Contrib. Mineral. Petrol., 164, 715–729, https://doi.org/10.1007/s00410-012-0772-2, 2012.
Taroev, V., Göttlicher, J., Kroll, H., Kashaev, A., Suvorova, L., Pentinghaus, H., Bernotat-Wulf, H., Breit, U., Tauson, V., and Lashkevich, V.: Synthesis and structural state of K-feldspars in the system K[AlSi3O8]-K[FeSi3O8], Eur. J. Mineral., 635–651, https://doi.org/10.1127/0935-1221/2008/0020-1840, 2008.
Tegner, C.: Iron in plagioclase as a monitor of the differentiation of the Skaergaard intrusion, Contrib. Mineral. Petrol., 128, 45–51, https://doi.org/10.1007/s004100050292, 1997.
Tegner, C. and Cawthorn, R. G.: Iron in plagioclase in the Bushveld and Skaergaard intrusions: implications for iron contents in evolving basic magmas, Contrib. Mineral. Petr., 159, 719–730, https://doi.org/10.1007/s00410-009-0450-1, 2010.
Thompson, D. (Ed.): A Guide to fossicking in the Northern Territory, 2nd Edn., Northern Territory Department of Mines and Energy, Darwin, 73 pp., ISBN 978-0-7245-0965-2, 1986.
Thompson Jr., J. B.: Chemical reactions in crystals, Am. Mineral., 54, 341–375, 1969.
van Aken, P. A. and Liebscher, B.: Quantification of ferrous/ferric ratios in minerals: new evaluation schemes of Fe L23 electron energy-loss near-edge spectra, Phys. Chem. Miner., 29, 188–200, https://doi.org/10.1007/s00269-001-0222-6, 2002.
Voll, G., Evangelakakis, C., and Kroll, H.: Revised two-feldspar geothermometry applied to Sri Lankan feldspars, Precambrian Res., 66, 351–377, https://doi.org/10.1016/0301-9268(94)90058-2, 1994.
Wen, S. and Nekvasil, H.: SOLVALC: An interactive graphics program package for calculating the ternary feldspar solvus and for two-feldspar geothermometry, Comput. Geosci., 20, 1025–1040, https://doi.org/10.1016/0098-3004(94)90039-6, 1994.
Wenk, H.-R., Chen, K., and Smith, R.: Morphology and microstructure of magnetite and ilmenite inclusions in plagioclase from Adirondack anorthositic gneiss, Am. Mineral., 96, 1316–1324, https://doi.org/10.2138/am.2011.3760, 2011.
Wilke, M., Farges, F., Petit, P.-E., Brown, G. E., and Martin, F.: Oxidation state and coordination of Fe in minerals: An Fe K-XANES spectroscopic study, Am. Mineral., 86, 714–730, https://doi.org/10.2138/am-2001-5-612, 2001.
Wones, D. R. and Appleman, D. E.: Properties of Synthetic Triclinic KFeSi3O8, Iron-microcline, with some Observations on the Iron-microclineIron-sanidine Transition, J. Petrol., 4, 131–137, https://doi.org/10.1093/petrology/4.1.131, 1963.
Xu, H., Jin, S., Lee, S., and Hobbs, W. C. F.: Nano-Phase KNa(Si6Al2)O16 in Adularia: A New Member in the Alkali Feldspar Series with Ordered K–Na Distribution, Minerals, 9, 649, https://doi.org/10.3390/min9110649, 2019.
Yazdi, M. B., Goyallon, M.-L., Bitsch, T., Kastner, A., Schlott, M., and Alff, L.: Transparent magnetic oxide thin films of Fe3O4 on glass, Thin Solid Films, 519, 2531–2533, https://doi.org/10.1016/j.tsf.2010.12.003, 2011.