Hochleitnerite, [K(H₂O)]Mn₂(Ti₂Fe)(PO₄)₄O₂(H₂O)₁₀  ⋅  4H₂O, a new paulkerrite-group mineral, from the Hagendorf-Süd pegmatite, Oberpfalz, Bavaria, Germany

Hochleitnerite, [K(H₂O)]Mn₂(Ti₂Fe)(PO₄)₄O₂(H₂O)₁₀ ⋅ 4H₂O, is a new paulkerrite-group mineral from the Hagendorf-Süd pegmatite, Oberpfalz, Bavaria, Germany. It was found in specimens of altered zwieselite, in association with fluorapatite, rockbridgeite, columbite and sub-micrometre rods of uranophane. Hochleitnerite occurs as isolated and intergrown pale-yellow, diamond-shaped tablets with thicknesses reaching 50 µm and lengths of 120 µm. The crystals are flattened on {010}, slightly elongated on [001], and bounded by the {111} and {010} forms. The calculated density is 2.40 g cm⁻³. Optically, hochleitnerite crystals are biaxial (+), with α= 1.615(2), β= 1.621(2) and γ= 1.645(2) (measured in white light). The calculated 2V is 53.8^∘. The empirical formula is [K(H₂O)](Mn${}_{\mathrm{1.51}}^{\mathrm{2}+}$Fe${}_{\mathrm{0.49}}^{\mathrm{2}+}$)_Σ2.00(Ti${}_{\mathrm{1.62}}^{\mathrm{4}+}$Fe${}_{\mathrm{0.19}}^{\mathrm{3}+}$Al_0.15)_Σ2.96(PO₄)_4.00[O_1.50F_0.23(OH)_0.27]_Σ2.00(H₂O)₁₀ ⋅ 4H₂O. Hochleitnerite has space group Pbca and unit-cell parameters a= 10.5513(3) Å, b= 20.6855(17) Å, c= 12.4575(4) Å, V= 2718.96(15) ų and Z= 4. The crystal structure was refined using single-crystal data to wR_obs= 0.082 for 2242 reflections with I > 3σ(I). The crystal structure contains corner-connected linear trimers of Ti-centred octahedra that share corners with PO₄ tetrahedra to form 10-member rings parallel to (010). K⁺ cations and water molecules are located within the rings. Additional corner sharing of the PO₄ tetrahedra with MnO₂(H₂O)₄ octahedra occurs along [010] to complete the 3D framework structure.

1 Introduction

Hochleitnerite was found by one of the authors (Erich Keck) in specimens of altered zweiselite that he collected in the 1970s at the 64 to 76 m level of the Hagendorf Süd pegmatite mine in the Oberpfalz, northeastern Bavaria, Germany (49^∘39^′1^′′ N, 12^∘27^′35^′′ E). It was identified by Erich Keck as a benyacarite-related mineral (Keck et al., 1998), and the specimen was forwarded in 2019 to CSIRO, Melbourne, for further characterisation as part of an ongoing Australian–Bavarian collaborative study on secondary phosphate minerals from the now defunct Hagendorf Süd pegmatite mine (Birch et al., 2018). Single-crystal diffraction studies combined with electron microprobe (EMP) analyses confirmed the mineral to be a new species, which was approved by the International Mineralogical Association (IMA) Commission on New Minerals, Nomenclature and Classification (CNMNC), IMA-2022-141.

The name honours Rupert Hochleitner (born 1954) for his important contributions to the crystal chemistry of minerals and the dissemination of knowledge on mineral identification through his books on the subject, translated into 12 languages. After completion of his doctoral thesis at the Institute of Crystallography and Applied Mineralogy, Ludwig Maximilian University, Munich, Rupert Hochleitner was chief editor of the mineralogical journal Lapis for 14 years. In 1993 he became vice director of the Mineralogical State Collection, Munich (SNSB), where he worked until his retirement in 2019. He is currently an honorary scientist at the Mineralogical State Collection. Rupert Hochleitner has published extensively on the application of Mössbauer, Raman and NMR spectroscopy to mineral characterisation. He has made major contributions to publications describing the characterisation of the Hagendorf Süd minerals keckite, Mn-bearing eleonorite, whiteite-(CaMnFe) and pleysteinite. Rupert Hochleitner has given his permission for the mineral name. The holotype specimen is housed in the mineralogical collections of the Natural History Museum of Los Angeles County, catalogue number 76 277. The Dana classification number for hochleitnerite is 43.11.21 (Gaines et al., 1997), defined as the paulkerrite group, which includes paulkerrite (Peacor et al., 1984), mantiennéite (Fransolet et al., 1984) and benyacarite (Demartin et al., 1993, 1997). The paulkerrite group has been approved by the IMA CNMNC, proposal 22-K-bis, with the addition of new members pleysteinite (Grey et al., 2023), hochleitnerite and rewitzerite (IMA 2023-005).

2 Occurrence and paragenesis

Hochleitnerite crystals occur on altered zwieselite (Fig. 1). The zwieselite, with composition Fe_1.2Mn_0.8(PO₄)F, is bleached to a pale-yellow colour and is microporous. Minerals closely associated with hochleitnerite and zwieselite are rockbridgeite, fluorapatite, columbite and sub-micrometre rods of uranophane. Other associated minerals are quartz and a jahnsite-group mineral.

Figure 1 Transparent crystals of hochleitnerite associated with colourless microcrystalline fluorapatite and black crystals of rockbridgeite. Field of view: 0.84 mm. Photo by Anthony Kampf.

Titanium-bearing minerals are very rare at Hagendorf Süd, with only biotite, ilmenite and a variety of uranpyrochlore recorded in Mindat. The most likely source of the Ti in hochleitnerite is the altered zwieselite matrix that it is in contact with. Chemical analyses of zwieselite in altered zwieselite specimens from five different locations at the Hagendorf pegmatite give TiO₂ contents in the range 0.08 wt % to 0.19 wt %. The presence of hochleitnerite crystals lining vugs formed by leaching of zwieselite suggests that hochleitnerite formed from zwieselite by dissolution–reprecipitation, and the presence of considerable H₂O in its formula indicates that this occurred at a relatively low temperature.

3 Physical and optical properties

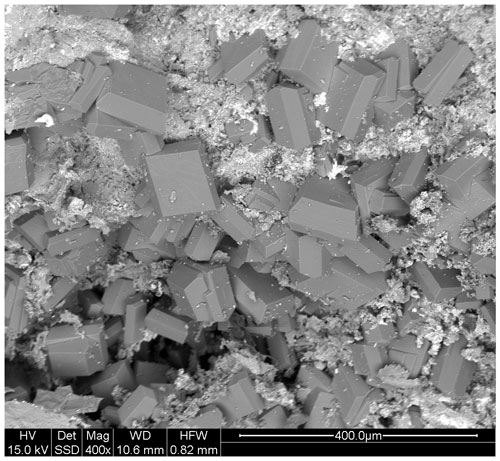
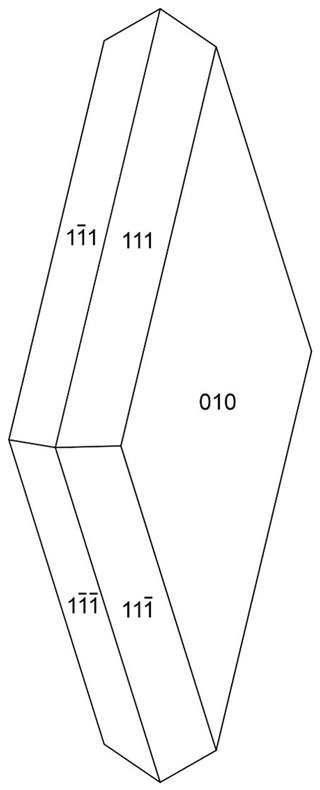
Hochleitnerite forms isolated and intergrown pale-yellow, diamond-shaped tablets on fine-grained zwieselite (Figs. 1 and 2). The crystals have dimensions of typically 20 to 80 µm. The crystals are flattened on {010}, slightly elongated on [001], and bounded by the {111} and {010} forms (Fig. 3). The calculated density, for the empirical formula and single-crystal cell volume, is 2.40 g cm⁻³.

Optically, hochleitnerite crystals are biaxial (+), with α= 1.615(2), β= 1.621(2) and γ= 1.645(2) (measured in white light). The calculated 2V is 53.8^∘; 2V could not be measured because of the presence of apparent sector zoning, which distorts the conoscopic figure and makes extinction observations unreliable. Dispersion was moderate with r < v, and pleochroism was not observed. The optical orientation is X= b, Y= c and Z= a. The Gladstone–Dale compatibility index (Mandarino, 1981) is 0.027 (excellent) based on the empirical formula and the calculated density.

Figure 2 Back-scattered electron image of hochleitnerite crystals on zwieselite.

Figure 3 Crystal drawing of hochleitnerite and clinographic projection in standard orientation.

4 Chemical composition

Crystals of hochleitnerite were analysed using wavelength-dispersive spectrometry on a JEOL JXA 8500F Hyperprobe operated at an accelerating voltage of 15 kV and a beam current of 2.2 nA. The beam was defocused to 10 µm. Analytical electron microprobe (EMP) results (average of 12 analyses on 12 different crystals) are given in Table 1. There was insufficient material for direct determination of H₂O, the presence of which was indicated by the low EMP analysis sum of oxides and confirmed by Raman spectroscopy, so it was based upon the crystal structure analysis with 15 H₂O per formula unit. The Fe²⁺ $/$ Fe³⁺ ratio was based on the crystal structure, with Fe²⁺ assigned with all other divalent elements (Mg and Mn²⁺) to the M1 site, and the remaining iron as Fe³⁺ was assigned to the M2 and M3 sites.

Table 1 Analytical data (wt%) for hochleitnerite.

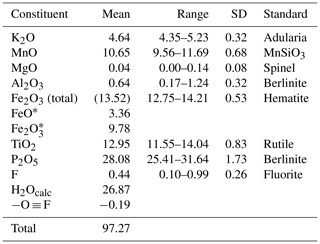
^* Fe²⁺ $/$ Fe³⁺ based on the crystal structure, with Fe²⁺ assigned with all Mn²⁺ and Mg at the M1 site and the remaining iron as Fe³⁺ assigned to the M2 and M3 sites.

The EMP results were normalised to 9 cations per formula unit and 33 (O + F), giving the atomic fractions K_1.00Mn_1.51Fe${}_{\mathrm{0.49}}^{\mathrm{2}+}$Fe${}_{\mathrm{1.19}}^{\mathrm{3}+}$Ti_1.62Al_0.15P_4.00O_32.77F_0.23H_30.27. The least-squares program OccQP (Wright et al., 2000) was used to optimise the site occupations for the M1 to M3 sites based on the refined site scattering and bond lengths from the crystal structure refinement combined with the chemical analyses, giving the following structural formula: ^A[(H₂O)_1.00K_1.00]_Σ2.00 ^M1(Mn${}_{\mathrm{1.51}}^{\mathrm{2}+}$Fe${}_{\mathrm{0.49}}^{\mathrm{2}+}$)_Σ2.00^M2(Ti${}_{\mathrm{1.08}}^{\mathrm{4}+}$Fe${}_{\mathrm{0.73}}^{\mathrm{3}+}$Al_0.15)_Σ1.96^M3(Ti${}_{\mathrm{0.54}}^{\mathrm{4}+}$ Fe${}_{\mathrm{0.46}}^{\mathrm{3}+}$)_Σ1.00(PO₄)_4.00[O_1.50F_0.23(OH)_0.27]_Σ2.00(H₂O)₁₀⋅4H₂O. The corresponding end-member formula is [H₂O]₂Mn₂Ti₃(PO₄)₄O₂(H₂O)₁₀ ⋅ 4H₂O.

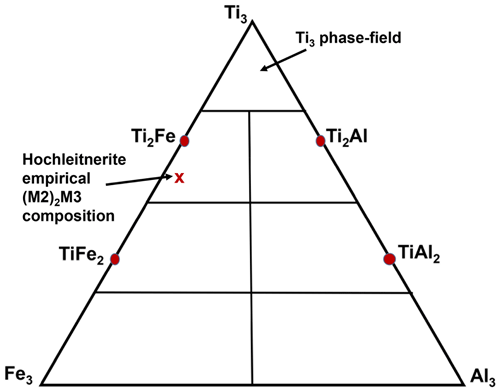
Although this formula was approved by the IMA CNMNC (IMA-2022-141), it was criticised by some voting members because it does not include K, which is co-dominant with H₂O at the A site. The problem arises because of almost complete mixing of Ti and Fe³⁺ at the crystalochemically similar M2 and M3 sites, so the constituent assignments at these two sites depend sensitively on the location of the minor Al. With Al located at the M3 site rather than at the M2 site, the program OccQP gives dominant Fe³⁺, rather than Ti at the M3 site, and leads to the alternative end-member formula [K(H₂O)]Mn₂Ti₂Fe(PO₄)₄O₂(H₂O)₁₀ ⋅ 4H₂O. To overcome the problem, common in paulkerrite group minerals, of very strong mixing of Fe³⁺ and Ti at the M2 and M3 sites, the compositions of these two sites were merged. The resulting empirical chemical formula can hence be rewritten as ^A[(H₂O)_1.00K_1.00]_Σ2.00^M1(Mn${}_{\mathrm{1.51}}^{\mathrm{2}+}$Fe${}_{\mathrm{0.49}}^{\mathrm{2}+}$)_Σ2.00^M2+M3(Ti${}_{\mathrm{1.62}}^{\mathrm{4}+}$Fe${}_{\mathrm{1.19}}^{\mathrm{3}+}$ Al_0.15)_Σ2.96(PO₄)_4.00[O_1.50F_0.23(OH)_0.27]_Σ2.00(H₂O)₁₀ ⋅ 4H₂O. This formula disregards the Ti–Fe³⁺ disordering over M2 and M3 and leads to the end-member formula [K(H₂O)]Mn₂(Ti₂Fe)(PO₄)₄O₂(H₂O)₁₀ ⋅ 4H₂O, which requires K₂O 4.79, MnO 14.44, Fe₂O₃ 8.13, TiO₂ 16.26, P₂O₅ 28.90 and H₂O 27.448, with a total of 100.00 wt %. This revised formula was approved by the IMA-CNMNC in a paulkerrite-group mineral nomenclature proposal (revised proposal 22-K-bis). The details of this nomenclature, based on site-total-charge method (Bosi et al., 2019), will be reported separately, but the result for the merged M2 and M3 site compositions is illustrated by the ternary diagram shown in Fig. 4. This diagram shows the possible end-member compositions based on the occupation of (M2)₂M3 by Al, Ti and Fe³⁺. The empirical composition of hochleitnerite, shown by the cross, is located in the compositional field for the end-member (Ti₂Fe).

Figure 4 Ternary diagram for (M2)₂M3 site Al–Ti–Fe³⁺ compositions, showing end-member compositions (Al₂Ti, AlTi₂, etc.) and the location of the experimental composition of hochleitnerite.

5 Raman spectroscopy

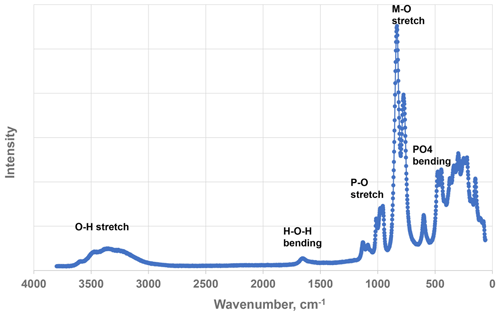
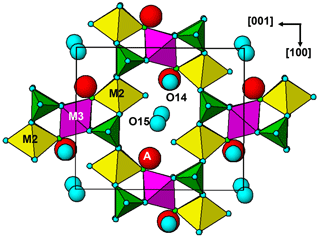
Raman spectroscopy was conducted on a Horiba XploRA PLUS spectrometer using a 532 nm diode laser, 100 µm slit, 1800 g mm⁻¹ diffraction grating and a 100× (0.9 NA) objective. The spectrum is shown in Fig. 5. The O–H stretching region has a broad peak with maxima at 3470, 3225, and 3305 cm⁻¹ and a sharp shoulder at 3580 cm⁻¹. The H–O–H bending mode region for water has a peak at 1660 cm⁻¹. The P–O stretching region has three peaks at 955, 975 and 1015 cm⁻¹ and two weaker peaks at 1080 and 1130 cm⁻¹. The three stronger peaks correspond to symmetric stretching modes, and the weaker peaks correspond to symmetric P–O stretching modes. Bending modes of the (PO₄)³⁻ groups are manifested by a band centred at 590 cm⁻¹ and a pair of bands at 440 and 470 cm⁻¹. Peaks at lower wavenumbers are related to lattice vibrations. The intense pair of peaks at 775 and 850 cm⁻¹ is an interesting feature of the spectrum. The tentative assignment of the peaks is to M–(O,F) stretching vibrations for short M–X bonds that occur in linear trimers of corner-connected octahedra M2–M3–M2 in the structure, where X is the bridging anion between the octahedra (Fig. 6). The potassium titanyl phosphate, KTiOPO₄, has chains of corner-connected octahedra with alternating short and long Ti–O bonds, and the Raman spectrum has an intense band at 770 cm⁻¹ that has been assigned to the symmetric Ti–O stretching vibration (Tu et al., 1996). Strong Raman bands in the range 800 to 900 cm⁻¹ have been reported and assigned to Ti–O stretch vibrations for several potassium titanium oxides, which have corner-connected TiO₆ octahedra involving short (∼ 1.8 Å) Ti–O bonds (Bamberger et al., 1990). The M2 site in hochleitnerite similarly has the M2 atom displaced from the centre of the octahedron towards the corner-sharing X anion with the M3-centred octahedron giving a short (1.806 Å) M2–(O,F) bond.

Figure 5 Raman spectrum for hochleitnerite.

Figure 6 The (010) slice of the hochleitnerite structure.

6 Crystallography

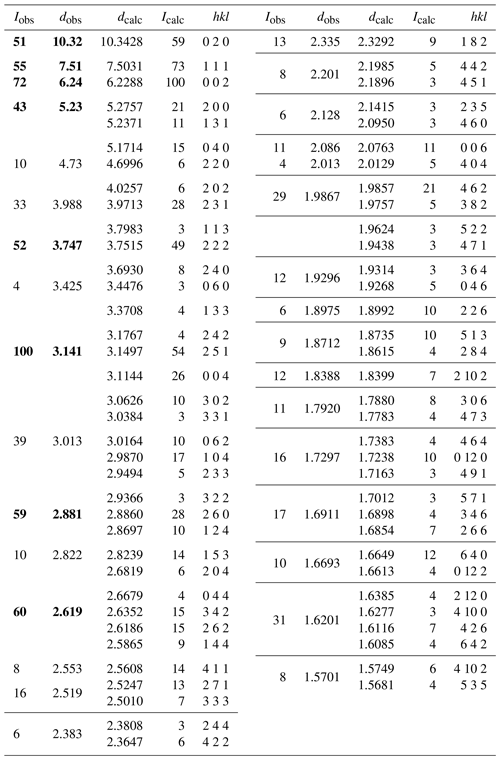
X-ray powder diffraction data were recorded using a Rigaku R-Axis Rapid II curved imaging plate microdiffractometer with monochromatised MoKα radiation. A Gandolfi-like motion on the ϕ and ω axes was used to randomise the sample. Observed d values and intensities were derived by profile fitting using JADE Pro software (Materials Data, Inc.). Data are given in Table 2. Refined orthorhombic unit-cell parameters (space group Pbca (#61)) are a= 10.557(10) Å, b= 20.710(18) Å, c= 12.502(11) Å, V= 2733.3(2) ų and Z= 4.

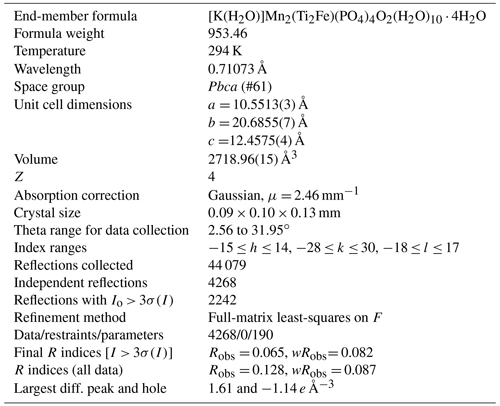
Single-crystal data were collected at 294 K using a XtaLAB Synergy four-circle diffractometer equipped with a Dualflex Hypix detector and using MoKα radiation, λ= 0.71073 Å. Refined unit-cell parameters and other data collection details are given in Table 3.

Table 2 Powder X-ray diffraction data (d in Å) for hochleitnerite (I_calc>2.5). Strongest reflections shown in bold.

Table 3 Crystal data and structure refinement for hochleitnerite.

Structure refinement

A structural model for hochleitnerite was obtained in space group Pbca using SHELXT (Sheldrick, 2015). It was found to be the same as that reported for benyacarite, (H₂O,K)₂M1₂M2₂Ti(PO₄)₄(O,F)₂ ⋅ 14H₂O (Demartin et al., 1993), where the M1 and M2 sites contain divalent and trivalent cations, respectively. Based on bond lengths, Mn²⁺ from the EMP analysis was assigned to the M1 site with Fe²⁺ completing the site filling. The scattering curves for Fe and Ti were used for the M2 and M3 sites, respectively, and a mix of K and O (for H₂O) was assigned to the A site. Refined site scattering values were used in the program OccQP to optimise site populations.

Refinement with anisotropic displacement parameters for all atoms in JANA2006 (Petříček et al., 2014) converged at R_obs= 0.065 for 2242 reflections with I > 3σ(I). An unambiguous location of H atoms in the correct configurations for H₂O molecules in difference Fourier maps was not achieved. Partial H positions have been previously reported for benyacarite (Demartin et al., 1993). Details of the data collection and refinement are given in Table 3. It is interesting to note that although the merging R_int factor was high (20 %) due to weak reflection intensities (mean $I/\mathit{\sigma }\left(I\right)=$ 2.9), the high redundancy in measured reflections of 10.3 resulted in averaged intensities that led to a reasonable refinement and sensible crystal chemistry of the model.

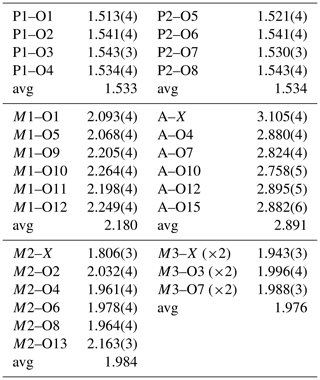
The refined coordinates, equivalent isotropic displacement parameters and bond valence sum (BVS) values (Gagné and Hawthorne, 2015) from the single-crystal refinement are reported in Table 4. Selected interatomic distances are reported in Table 5. Although the H atoms were not located, the BVS values in Table 4 clearly show the presence of seven independent H₂O groups, O9 to O15.

Table 4 Atom coordinates, equivalent isotropic displacement parameters (Ų) and bond valence sums (BVS, in valence units) for hochleitnerite.

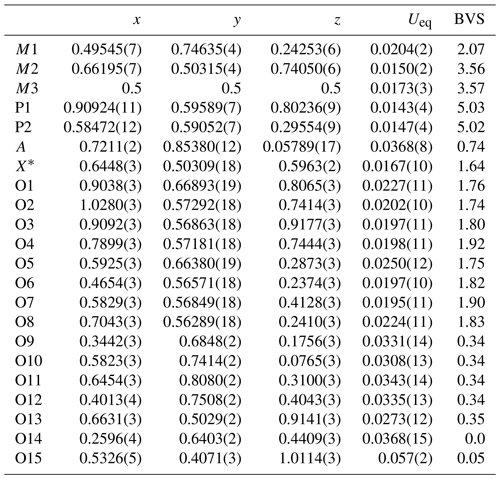
Site scattering (electrons): M1 = 25.25; M2 = 22.34; M3 =  23.84. A site occupancy = K_0.438(9)O_0.562(9). X^* denotes the bridging anion site between M2- and M3-centred octahedra.

Table 5 Polyhedral bond lengths [Å] for hochleitnerite.

Figure 7 The (001) slice through the structure of hochleitnerite.

Figure 8 The (11-2) layer in the crystal structure for titanite, showing same heteropolyhedral topology as for the (001) layer in hochleitnerite (Fig. 7).

7 Discussion

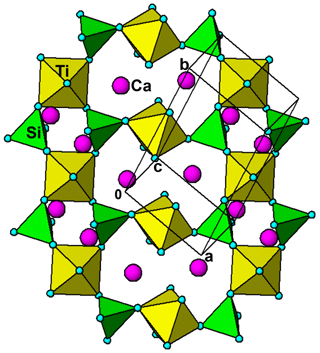
Hochleitnerite is isostructural with benyacarite (Demartin et al., 1993, 1997) and the recently described Al analogue pleysteinite (Grey et al., 2023). A key feature of the structure is the presence of linear trimers of corner-connected octahedra M2–M3–M2, which are oriented approximately parallel to [102] and [−102] as shown in Fig. 6. The M2- and M3-centred octahedra have compositions M2O₄X(H₂O) and M3O₄X₂, respectively, where O anions are shared with PO₄ tetrahedra and X is the bridging anion between the octahedra. The M2-centred octahedron is characterised by a displacement of M2 towards X to give a short M2–X distance of 1.80 Å and a much longer opposing M2–H₂O distance of 2.16Å. The octahedral distortion is similar to that occurring in compounds containing the titanyl ion such as KTiO(PO₄) and gives rise to a strong Raman band near 800 cm⁻¹ as discussed in the Raman spectroscopy section. Such a distortion is not possible for the M3-centred octahedron, since it is located at an inversion centre and has three pairs of equivalent anions, but the two M3–X distances are considerably shorter than the four M3–O distances. The octahedral trimers are interconnected via corner sharing with PO₄ tetrahedra to form (010) heteropolyhedral layers shown in Fig. 6. The corner-connected octahedra and tetrahedra form 10-member rings that border cavities containing the A-site species (K⁺ and H₂O) and water molecules (O14 and O15 in Table 4). The water molecule O15 is coordinated to K at the A site at a distance of 2.88 Å, while O14 only has H-bonded contacts.

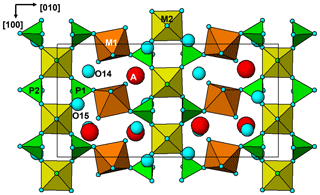
The (010) heteropolyhedral layers are interconnected along [010] into a 3D framework via M1-centred octahedra of composition M1O₂(H₂O)₄. These octahedra share trans O anions with PO₄ tetrahedra as shown in Fig. 7. This view of the structure, an (001) slice, shows that the M2-centred octahedra and PO₄ tetrahedra form M2(PO₄)₂X(H₂O) kröhnkite-type ribbons (Hawthorne, 1985) along [100]. The ribbon repeat distance along [100] of 10.55 Å in hochleitnerite is double that in kröhnkite due to the tetrahedra in successive four-member rings having their apices pointing in opposite directions along [001]. It is interesting to note that the heteropolyhedral layer in Fig. 7, containing four-member and eight-member rings, has topological equivalents in GeOHPO₄-type structures that include minerals such as kieserite (MgSO₄ ⋅ H₂O), titanite (CaTiOSiO₄), lazulite (MgAl₂(PO₄)₂(OH)₂) and amblygonite ((Li,Na)Al(PO₄)(F,OH)) (Mayer and Völlenkle, 1972). The example of titanite is shown in Fig. 8 where the close heteropolyhedral layer relationship with hochleitnerite extends also to the Ca atoms, which occupy similar positions in the eight-member rings to the A-site cations in hochleitnerite. Other minerals that have the same topology of kröhnkite-type ribbons bridged by MO₂(H₂O)₄ octahedra as occurs in hochleitnerite (Fig. 7) include laueite-group minerals and geometrical isomers like stewartite (Krivovichev, 2004).